Difference between revisions of "2006 Cyprus MO/Lyceum/Problem 11"
(→Solution) |
I like pie (talk | contribs) (Standardized answer choices) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
==Problem== | ==Problem== | ||
| − | + | [[Image:2006 CyMO-11.PNG|250px|right]] | |
| − | [[Image:2006 CyMO-11.PNG|250px]] | ||
| − | |||
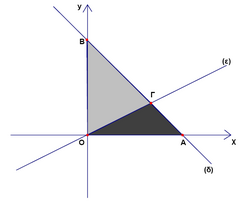
The lines <math>(\epsilon):x-2y=0</math> and <math>(\delta):x+y=4</math> intersect at the point <math>\Gamma</math>. If the line <math>(\delta)</math> intersects the axes <math>Ox</math> and <math>Oy</math> to the points <math>A</math> and <math>B</math> respectively, then the ratio of the area of the triangle <math>OA\Gamma</math> to the area of the triangle <math>OB\Gamma</math> equals | The lines <math>(\epsilon):x-2y=0</math> and <math>(\delta):x+y=4</math> intersect at the point <math>\Gamma</math>. If the line <math>(\delta)</math> intersects the axes <math>Ox</math> and <math>Oy</math> to the points <math>A</math> and <math>B</math> respectively, then the ratio of the area of the triangle <math>OA\Gamma</math> to the area of the triangle <math>OB\Gamma</math> equals | ||
| − | + | <math>\mathrm{(A)}\ \frac{1}{3}\qquad\mathrm{(B)}\ \frac{2}{3}\qquad\mathrm{(C)}\ \frac{3}{5}\qquad\mathrm{(D)}\ \frac{1}{2}\qquad\mathrm{(E)}\ \frac{4}{9}</math> | |
| − | |||
| − | B | ||
| − | |||
| − | C | ||
| − | |||
| − | D | ||
| − | |||
| − | E | ||
==Solution== | ==Solution== | ||
| Line 25: | Line 15: | ||
<math>B=(0,4)</math> | <math>B=(0,4)</math> | ||
| − | <math>\Gamma =(\frac{8}{3},\frac{4}{3})</math> | + | <math>\Gamma =\left(\frac{8}{3},\frac{4}{3}\right)</math> |
We find the area of triangles: | We find the area of triangles: | ||
| Line 39: | Line 29: | ||
==See also== | ==See also== | ||
{{CYMO box|year=2006|l=Lyceum|num-b=10|num-a=12}} | {{CYMO box|year=2006|l=Lyceum|num-b=10|num-a=12}} | ||
| + | |||
| + | [[Category:Introductory Geometry Problems]] | ||
Latest revision as of 09:37, 27 April 2008
Problem
The lines ![]() and
and ![]() intersect at the point
intersect at the point ![]() . If the line
. If the line ![]() intersects the axes
intersects the axes ![]() and
and ![]() to the points
to the points ![]() and
and ![]() respectively, then the ratio of the area of the triangle
respectively, then the ratio of the area of the triangle ![]() to the area of the triangle
to the area of the triangle ![]() equals
equals
![]()
Solution
We find some coordinates:
![]()
![]()
![]()
![]()
We find the area of triangles:
![]()
![]()
![]()
![]()
See also
| 2006 Cyprus MO, Lyceum (Problems) | ||
| Preceded by Problem 10 |
Followed by Problem 12 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 • 26 • 27 • 28 • 29 • 30 | ||