Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1987 AIME Problems/Problem 4"

m (→‎See also)
m (→‎Solution 2)
 
(12 intermediate revisions by 10 users not shown)
Line 1: Line 1:
 
== Problem ==
 
== Problem ==
 +
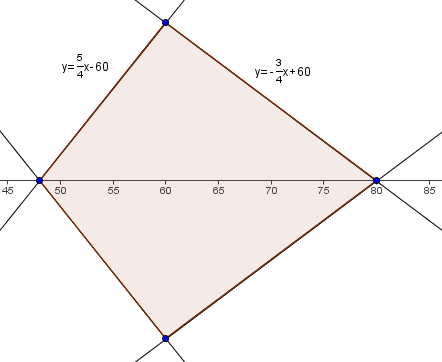
Find the [[area]] of the region enclosed by the [[graph]] of <math>|x-60|+|y|=\left|\frac{x}{4}\right|.</math>
  
−
== Solution ==
+
== Solution 1 ==
 +
[[Image:1987_AIME-4.png]]
 +
 
 +
Since <math>|y|</math> is [[nonnegative]], <math>\left|\frac{x}{4}\right| \ge |x - 60|</math>. Solving this gives us two equations: <math>\frac{x}{4} \ge x - 60\ \mathrm{and} \ -\frac{x}{4} \le x - 60</math>. Thus, <math>48 \le x \le 80</math>. The [[maximum]] and [[minimum]] y value is when <math>|x - 60| = 0</math>, which is when <math>x = 60</math> and <math>y = \pm 15</math>. Since the graph is [[symmetry|symmetric]] about the y-axis, we just need [[casework]] upon <math>x</math>. <math>\frac{x}{4} > 0</math>, so we break up the condition <math>|x-60|</math>:
 +
 
 +
*<math>x - 60 > 0</math>. Then <math>y = -\frac{3}{4}x+60</math>.
 +
*<math>x - 60 < 0</math>. Then <math>y = \frac{5}{4}x-60</math>.
 +
 
 +
The area of the region enclosed by the graph is that of the quadrilateral defined by the points <math>(48,0),\ (60,15),\ (80,0), \ (60,-15)</math>. Breaking it up into triangles and solving or using the [[Shoelace Theorem]], we get <math>2 \cdot \frac{1}{2}(80 - 48)(15) = \boxed{480}</math>.
 +
 
 +
== Solution 2 ==
 +
 
 +
Since <math>|y|</math> is the only present <math>y</math> "term" in this equation, we know that the area must be symmetrical about the x-axis.
 +
 
 +
We'll consider the area when <math>y>0</math> and we only consider the portion enclosed with <math>y=0</math>. Then, we'll double that area since the graph is symmetrical.
 +
 
 +
Now, let us remove the absolute values:
 +
 
 +
When <math>x\ge 60</math>: <math>x-60+y=0.25x</math>. This rearranges to <math>y=-0.75x+60</math>.
 +
 
 +
When <math>0\le x<60</math>: <math>60-x+y=0.25</math>. So <math>y=1.25x-60</math>.
 +
 
 +
When <math>x<0</math>: <math>60-x+y=-0.25x</math>. So <math>y=0.75x-60</math>.
 +
 
 +
By simple sketching, we see the shape that looks like the image in Solution 1 (graph it out and you'll see). We see that the partial area we seek in this part is the triangle with the vertices <math>(60,15)</math>, <math>(48,0)</math>, <math>(80,0)</math>. This triangle has an area of <math>(80-48)*15*0.5=240</math>.
 +
 
 +
Simply double the area and we get <math>\boxed{480}</math> as our final answer.
 +
~hastapasta
  
 
== See also ==
 
== See also ==
−
* [[1987 AIME Problems]]
+
{{AIME box|year=1987|num-b=3|num-a=5}}
  
−
{{AIME box|year=1987|num-b=3|num-a=5}}
+
[[Category:Intermediate Algebra Problems]]
 +
{{MAA Notice}}

Latest revision as of 16:25, 13 February 2024

Problem

Find the area of the region enclosed by the graph of $|x-60|+|y|=\left|\frac{x}{4}\right|.$

Solution 1

1987 AIME-4.png

Since $|y|$ is nonnegative, $\left|\frac{x}{4}\right| \ge |x - 60|$. Solving this gives us two equations: $\frac{x}{4} \ge x - 60\ \mathrm{and} \ -\frac{x}{4} \le x - 60$. Thus, $48 \le x \le 80$. The maximum and minimum y value is when $|x - 60| = 0$, which is when $x = 60$ and $y = \pm 15$. Since the graph is symmetric about the y-axis, we just need casework upon $x$. $\frac{x}{4} > 0$, so we break up the condition $|x-60|$:

  • $x - 60 > 0$. Then $y = -\frac{3}{4}x+60$.
  • $x - 60 < 0$. Then $y = \frac{5}{4}x-60$.

The area of the region enclosed by the graph is that of the quadrilateral defined by the points $(48,0),\ (60,15),\ (80,0), \ (60,-15)$. Breaking it up into triangles and solving or using the Shoelace Theorem, we get $2 \cdot \frac{1}{2}(80 - 48)(15) = \boxed{480}$.

Solution 2

Since $|y|$ is the only present $y$ "term" in this equation, we know that the area must be symmetrical about the x-axis.

We'll consider the area when $y>0$ and we only consider the portion enclosed with $y=0$. Then, we'll double that area since the graph is symmetrical.

Now, let us remove the absolute values:

When $x\ge 60$: $x-60+y=0.25x$. This rearranges to $y=-0.75x+60$.

When $0\le x<60$: $60-x+y=0.25$. So $y=1.25x-60$.

When $x<0$: $60-x+y=-0.25x$. So $y=0.75x-60$.

By simple sketching, we see the shape that looks like the image in Solution 1 (graph it out and you'll see). We see that the partial area we seek in this part is the triangle with the vertices $(60,15)$, $(48,0)$, $(80,0)$. This triangle has an area of $(80-48)*15*0.5=240$.

Simply double the area and we get $\boxed{480}$ as our final answer. ~hastapasta

See also

1987 AIME (Problems • Answer Key • Resources)
Preceded by
Problem 3 		Followed by
Problem 5
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1987_AIME_Problems/Problem_4&oldid=215442"