Difference between revisions of "Angle bisector"
| Line 1: | Line 1: | ||
| + | {{WotWAnnounce|week=June 6-12}} | ||
| + | |||
For an [[angle]] <math>\angle ABC</math>, the angle bisector of <math>\angle ABC</math> is the line from B such that the angle between this line and <math>BC</math> is equal to the angle between this line and <math>AB</math>. | For an [[angle]] <math>\angle ABC</math>, the angle bisector of <math>\angle ABC</math> is the line from B such that the angle between this line and <math>BC</math> is equal to the angle between this line and <math>AB</math>. | ||
Revision as of 20:41, 5 June 2008
| This is an AoPSWiki Word of the Week for June 6-12 |
For an angle 
, the angle bisector of is the line from B such that the angle between this line and 
is equal to the angle between this line and 
.
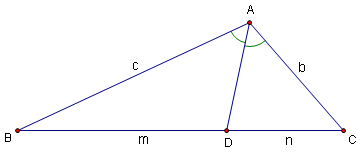
Features of Angle Bisectors
In a triangle, the angle bisectors (which are cevians) will all intersect at the incenter of the triangle.
See also
This article is a stub. Help us out by expanding it.
