Angle Bisector Theorem

Introduction

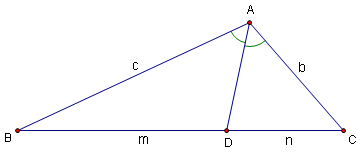
The Angle Bisector Theorem states that given triangle and angle bisector AD, where D is on side BC, then .

Proof

Method 1

Because of the ratios and equal angles in the theorem, we think of similar triangles. There are not any similar triangles in the figure as it now stands, however. So we think to draw in a carefully chosen line or two. Extending AD until it hits the line through C parallel to AB does just the trick as we shall see!

Since AB and CE are parallel, we know that and . Triangle ACE is isosceles meaning that AC = CE.

By AA, . By the properties of similar triangles, we arrive at our desired result:

Method 2

Let . Now, we can express the area of triangle ABD in two ways:

Thus .

Likewise, triangle ACD can be expressed in two different ways:

Thus .

But and since . Therefore, we can substitute back into our previous equation to get .

We conclude that , which was what we wanted.

See also

• Angle bisector
• Geometry
• Stewart's Theorem