1987 AIME Problems/Problem 9

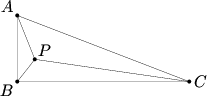
Problem

Triangle $\displaystyle ABC$ has right angle at $\displaystyle B$ , and contains a point $\displaystyle P$ for which $\displaystyle PA = 10$ , $\displaystyle PB = 6$ , and $\displaystyle \angle APB = \angle BPC = \angle CPA$ . Find $\displaystyle PC$ .

Solution

Let $PC = x$ .

Since the three angles $\angle APB$ , $\angle BPC$ and $\angle CPA$ are equal, each of them is equal to $120^\circ$ . By the Law of Cosines applied to triangles $\triangle APB$ , $\triangle BPC$ and $\triangle CPA$ at their respective angles $P$ , remembering that $\cos 120^\circ = -\frac12$ , we have

$AB^2 = 36 + 100 + 60 = 196$ , $BC^2 = 36 + x^2 + 6x$ and $CA^2 = 100 + x^2 + 10x$ .

Then by the Pythagorean Theorem, $AB^2 + BC^2 = CA^2$ so that

$x^2 + 10x + 100 = x^2 + 6x + 36 + 196$

and

$4x = 132$ so $x = 033$ .

1987 AIME (Problems • Answer Key • Resources)
Preceded by Problem 8	Followed by Problem 10
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

Page

Toolbox

Search

1987 AIME Problems/Problem 9

Problem

Solution

See also