2002 AMC 12B Problems/Problem 25
Contents
[hide]Problem
Let ![]() , and let
, and let ![]() denote the set of points
denote the set of points ![]() in the coordinate plane such that
in the coordinate plane such that
![]() The area of
The area of ![]() is closest to
is closest to
![]()
Solution 1
The first condition gives us that
![]()
which is a circle centered at ![]() with radius
with radius ![]() . The second condition gives us that
. The second condition gives us that
![]()
Thus either
![]()
or
![]()
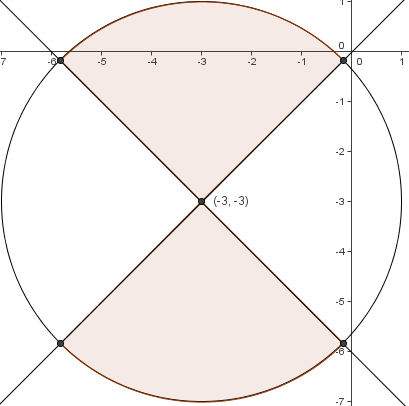
Each of those lines passes through ![]() and has slope
and has slope ![]() , as shown above. Therefore, the area of
, as shown above. Therefore, the area of ![]() is half of the area of the circle, which is
is half of the area of the circle, which is ![]() .
~SHEN KISLAY KAI
.
~SHEN KISLAY KAI
Solution 2
Similar to Solution 1, we proceed to get the area of the circle satisfying ![]() , or
, or ![]() .
.
Since ![]() , we have that by symmetry, if
, we have that by symmetry, if ![]() is in
is in ![]() , then
, then ![]() is not, and vice versa. Therefore, the shaded part of the circle above the line
is not, and vice versa. Therefore, the shaded part of the circle above the line ![]() has the same area as the unshaded part below
has the same area as the unshaded part below ![]() , and the unshaded part above
, and the unshaded part above ![]() has the same area as the shaded part below
has the same area as the shaded part below ![]() . This means that exactly half the circle is shaded, allowing us to divide by two to get
. This means that exactly half the circle is shaded, allowing us to divide by two to get ![]() . ~samrocksnature + ddot1 +Shen kislay kai
. ~samrocksnature + ddot1 +Shen kislay kai
See also
| 2002 AMC 12B (Problems • Answer Key • Resources) | |
| Preceded by Problem 24 |
Followed by Last problem |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()