Difference between revisions of "2022 AMC 8 Problems/Problem 25"
MRENTHUSIASM (talk | contribs) (→Solution 1 (Casework)) |
MRENTHUSIASM (talk | contribs) (Prioritized casework solutions.) |
||
| Line 28: | Line 28: | ||
~MRENTHUSIASM | ~MRENTHUSIASM | ||
| − | ==Solution 2 | + | ==Solution 2 (Casework)== |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
We can label the leaves as shown: | We can label the leaves as shown: | ||
| Line 67: | Line 59: | ||
~mahaler | ~mahaler | ||
| + | |||
| + | ==Solution 3 (Recursion)== | ||
| + | |||
| + | Denote <math>P_n</math> to be the probability that the cricket would return back to the first point after <math>n</math> hops. Then, we get the recursive formula <cmath>P_n = \frac13(1-P_{n-1})</cmath> because if the leaf is not on the target leaf, then there is a <math>\frac13</math> probability that it will make it back. | ||
| + | |||
| + | With this formula and the fact that <math>P_0=0,</math> we have <cmath>P_1 = \frac13, P_2 = \frac29, P_3 = \frac7{27},</cmath> so our answer is <math>\boxed{\textbf{(E) }\frac{7}{27}}</math>. | ||
| + | |||
| + | ~wamofan | ||
==See Also== | ==See Also== | ||
{{AMC8 box|year=2022|num-b=24|after=Last Problem}} | {{AMC8 box|year=2022|num-b=24|after=Last Problem}} | ||
{{MAA Notice}} | {{MAA Notice}} | ||
Revision as of 15:37, 29 January 2022
Contents
[hide]Problem
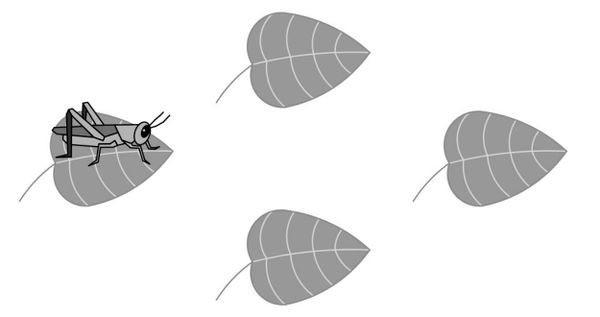
A cricket randomly hops between ![]() leaves, on each turn hopping to one of the other
leaves, on each turn hopping to one of the other ![]() leaves with equal probability. After
leaves with equal probability. After ![]() hops what is the probability that the cricket has returned to the leaf where it started?
hops what is the probability that the cricket has returned to the leaf where it started?
![]()
Solution 1 (Casework)
Let ![]() denote the leaf where the cricket starts and
denote the leaf where the cricket starts and ![]() denote one of the other
denote one of the other ![]() leaves. Note that:
leaves. Note that:
- If the cricket is at
 then the probability that it hops to
then the probability that it hops to  next is
next is 
- If the cricket is at
 then the probability that it hops to
then the probability that it hops to  next is
next is 
- If the cricket is at
 then the probability that it hops to
then the probability that it hops to  next is
next is 
We apply casework to the possible paths of the cricket:

The probability for this case is


The probability for this case is

Together, the probability that the cricket returns to ![]() is
is ![]()
~MRENTHUSIASM
Solution 2 (Casework)
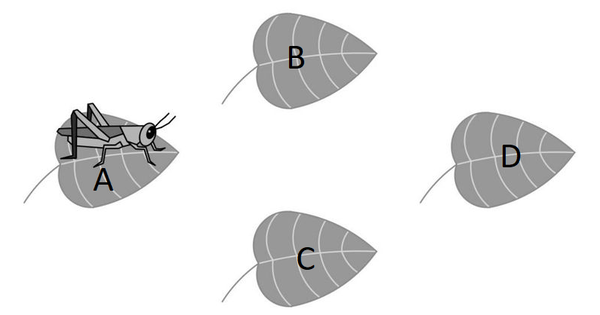
We can label the leaves as shown:
Carefully counting cases, we see that there are ![]() ways for the cricket to return to leaf
ways for the cricket to return to leaf ![]() after four hops if its first hop was to leaf
after four hops if its first hop was to leaf ![]() :
:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Taking advantage of symmetry, this also means there are ![]() ways if the cricket's first hop was to leaf
ways if the cricket's first hop was to leaf ![]() .
.
Finally, if the cricket's first hop was to leaf ![]() , we see that there are also
, we see that there are also ![]() ways:
ways:
![]()
![]()
![]()
![]()
![]()
![]()
![]()
So, in total, there are ![]() ways for the cricket to return to leaf
ways for the cricket to return to leaf ![]() after four hops.
after four hops.
Since there are ![]() possible ways altogether for the cricket to hop to any other leaf four times, the answer is
possible ways altogether for the cricket to hop to any other leaf four times, the answer is ![]() .
.
~mahaler
Solution 3 (Recursion)
Denote ![]() to be the probability that the cricket would return back to the first point after
to be the probability that the cricket would return back to the first point after ![]() hops. Then, we get the recursive formula
hops. Then, we get the recursive formula ![]() because if the leaf is not on the target leaf, then there is a
because if the leaf is not on the target leaf, then there is a ![]() probability that it will make it back.
probability that it will make it back.
With this formula and the fact that ![]() we have
we have ![]() so our answer is
so our answer is ![]() .
.
~wamofan
See Also
| 2022 AMC 8 (Problems • Answer Key • Resources) | ||
| Preceded by Problem 24 |
Followed by Last Problem | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AJHSME/AMC 8 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()