Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2015 AMC 10A Problems/Problem 19"

(I added a new solution)
(→‎Problem)
Line 1: Line 1:
 
==Problem==
 
==Problem==
  
−
The isosceles right triangle <math>ABC</math> has right angle at <math>C</math> and area <math>12.5</math>. The rays trisecting <math>\angle ACB</math> intersect <math>AB</math> at <math>D</math> and <math>E</math>. What is the area of <math>\bigtriangleup CDE</math>?
+
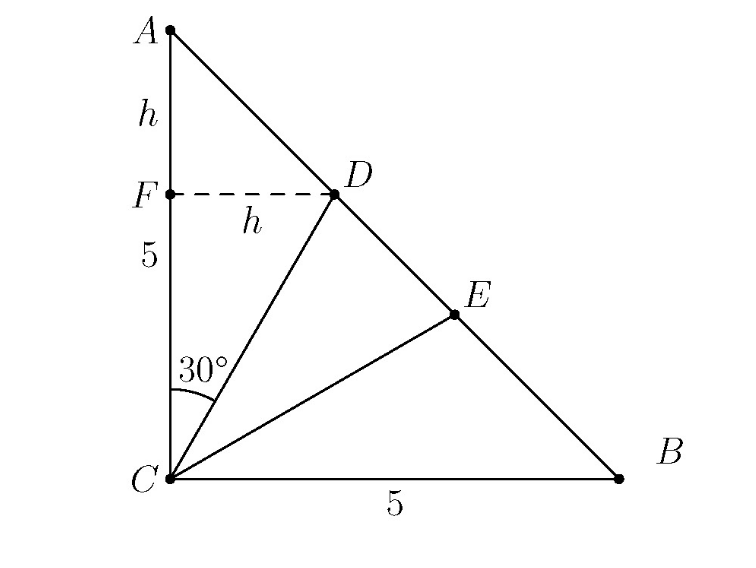
What is the ratio of the area of triangle <math>BDC</math> to the area of triangle <math>ADC</math>? Express your answer as a common fraction
  
 
<math> \textbf{(A) }\dfrac{5\sqrt{2}}{3}\qquad\textbf{(B) }\dfrac{50\sqrt{3}-75}{4}\qquad\textbf{(C) }\dfrac{15\sqrt{3}}{8}\qquad\textbf{(D) }\dfrac{50-25\sqrt{3}}{2}\qquad\textbf{(E) }\dfrac{25}{6} </math>
 
<math> \textbf{(A) }\dfrac{5\sqrt{2}}{3}\qquad\textbf{(B) }\dfrac{50\sqrt{3}-75}{4}\qquad\textbf{(C) }\dfrac{15\sqrt{3}}{8}\qquad\textbf{(D) }\dfrac{50-25\sqrt{3}}{2}\qquad\textbf{(E) }\dfrac{25}{6} </math>

Revision as of 20:27, 13 May 2024

Problem

What is the ratio of the area of triangle $BDC$ to the area of triangle $ADC$? Express your answer as a common fraction

$\textbf{(A) }\dfrac{5\sqrt{2}}{3}\qquad\textbf{(B) }\dfrac{50\sqrt{3}-75}{4}\qquad\textbf{(C) }\dfrac{15\sqrt{3}}{8}\qquad\textbf{(D) }\dfrac{50-25\sqrt{3}}{2}\qquad\textbf{(E) }\dfrac{25}{6}$

Solution 1 (No Trigonometry)

2015AMC10AProblem19Picture.png

$\triangle ADC$ can be split into a $45-45-90$ right triangle and a $30-60-90$ right triangle by dropping a perpendicular from $D$ to side $AC$. Let $F$ be where that perpendicular intersects $AC$.

Because the side lengths of a $45-45-90$ right triangle are in ratio $a:a:a\sqrt{2}$, $DF = AF$.

Because the side lengths of a $30-60-90$ right triangle are in ratio $a:a\sqrt{3}:2a$ and $AF + FC = 5$, $DF = \frac{5 - AF}{\sqrt{3}}$.

Setting the two equations for $DF$ equal to each other, $AF = \frac{5 - AF}{\sqrt{3}}$.

Solving gives $AF = DF = \frac{5\sqrt{3} - 5}{2}$.

The area of $\triangle ADC = \frac12 \cdot DF \cdot AC = \frac{25\sqrt{3} - 25}{4}$.

$\triangle ADC$ is congruent to $\triangle BEC$, so their areas are equal.

A triangle's area can be written as the sum of the figures that make it up, so $[ABC] = [ADC] + [BEC] + [CDE]$.

$\frac{25}{2} = \frac{25\sqrt{3} - 25}{4} + \frac{25\sqrt{3} - 25}{4} + [CDE]$.

Solving gives $[CDE] = \frac{50 - 25\sqrt{3}}{2}$, so the answer is $\boxed{\textbf{(D) }\frac{50 - 25\sqrt{3}}{2}}$

Note

Another way to get $DF$ is that you assume $AF=DF$ to be equal to $a$, as previously mentioned, and $CF$ is equal to $a\sqrt{3}$. $AF+DF=5=a+a\sqrt{3}$

Solution 2 (Trigonometry)

The area of $\triangle ABC$ is $12.5$, and so the leg length of $45 - 45 - 90$ $\triangle ABC$ is $5.$ Thus, the altitude to hypotenuse $AB$, $CF$, has length $\dfrac{5}{\sqrt{2}}$ by $45 - 45 - 90$ right triangles. Now, it is clear that $\angle{ACD} = \angle{BCE} = 30^\circ$, and so by the Exterior Angle Theorem, $\triangle{CDE}$ is an isosceles $30 - 75 - 75$ triangle. Thus, $DF = CF \tan 15^\circ = \dfrac{5}{\sqrt{2}} (2 - \sqrt{3})$ by the Half-Angle formula, and so the area of $\triangle CDE$ is $DF \cdot CF = \dfrac{25}{2} (2 - \sqrt{3})$. The answer is thus $\boxed{\textbf{(D) } \frac{50 - 25\sqrt{3}}{2}}$

Solution 3 (Analytical Geometry)

Because the area of triangle $ABC$ is $12.5$, and the triangle is right and isosceles, we can quickly see that the leg length of the triangle $ABC$ is 5. If we put the triangle on the coordinate plane, with vertex $C$ at the origin, and the hypotenuse in the first quadrant, we can use slope-intercept form and tangents to get three lines that intersect at the origin, $D$, and $E$. Then, you can use the distance formula to get the length of $DE$. The height is just $\frac{5}{\sqrt{2}}$, so the area is just $DE \cdot \frac{5}{\sqrt{2}} \cdot \frac{1}{2}=\boxed{\textbf{(D) } \frac{50 - 25\sqrt{3}}{2}}$


Solution 4 (Trigonometry)

Just like with Solution 1, we drop a perpendicular from $D$ onto $AC$, splitting it into a $30$-$60$-$90$ triangle and a $45$-$45$-$90$ triangle. We find that $AF=\frac{5\sqrt{3}-5}{2}$.

Now, since $\triangle AEC\cong \triangle BDC$ by ASA, $CE=CD$. Since, $DF=\frac{5\sqrt{3}-5}{2}$, $DC=2\cdot \frac{5\sqrt{3}-5}{2}=5\sqrt{3}-5$. By the sine area formula, $[CDE]=\frac{1}{2}\cdot \sin 30\cdot CD^2=\frac{1}{4}\cdot (100-50\sqrt{3})=\frac{50-25\sqrt{3}}{2}\implies \boxed{\textbf{(D)}}$

Solution 5 (Basic Trigonometry)

Prerequisite knowledge for this solution: the side ratios of a 30-60-90, and 45-45-90 right triangle.


We let point C be the origin. Since $\overline{CD}$ and $\overline{CE}$ trisect $\angle ACB = 90^{\circ}$, this means $m\angle CEB = 30^{\circ}$ and the equation of $\overline{CE}$ is $y=\frac{\sqrt{3}}{3}$ (you can figure out that the tangent of 30 degrees gives $\frac{\sqrt{3}}{3}$). Next, we can find A to be at $(0, 5)$ and B at $(5, 0)$, so the equation of $\overline{AB}$ is $y=-x+5$. So we have the system:

\[\begin{cases}y=\frac{\sqrt{3}}{3}x\\y=-x+5\end{cases}\]

By substituting values, we can arrive at $\frac{3+\sqrt{3}}{3}x=5$, or $x=5\cdot\frac{3}{3+\sqrt{3}}=\frac{15}{3+\sqrt{3}}$. We multiply $x=\frac{15}{3+\sqrt{3}}\cdot\frac{3-\sqrt{3}}{3-\sqrt{3}}=\frac{45-15\sqrt{3}}{6}=\frac{15-5\sqrt{3}}{2}$.

Dropping an altitude from E onto $\overline{CB}$, and calling the intersection point G, we find that $\triangle EGB$ is a 45-45-90 triangle with a leg of $\frac{15-5\sqrt{3}}{2}\cdot\frac{\sqrt{3}}{3}=\frac{15\sqrt{3}-15}{6}=\frac{5\sqrt{3}-5}{2}$. Thus, $EB=\frac{5\sqrt{3}-5}{2}\sqrt{2}=\frac{5\sqrt{6}-5\sqrt{2}}{2}$.

Dropping an altitude from C onto $\overline{AB}$, and calling the intersection point H, we find that $CH=\frac{5\sqrt{2}}{2}=BH$, and by the theorem of betweenness applied to H, E, and B, we get $HE=HB-EB=\frac{5\sqrt{2}}{2}-\frac{5\sqrt{6}-5\sqrt{2}}{2}=\frac{10\sqrt{2}-5\sqrt{6}}{2}$.

We are almost done. By symmetry, $HD=HE$, so to find the area of the triangle CED, we only need to multiply HE by CH, $\frac{10\sqrt{2}-5\sqrt{6}}{2}\cdot\frac{5\sqrt{2}}{2}=\frac{100-50\sqrt{3}}{4}=\frac{50-25\sqrt{3}}{2}$. This is answer choice $\boxed{\textbf{(D) } \frac{50-25\sqrt{3}}{2}}$ ~JH. L

Solution 6 (Law of Sines)

We know that the area of the right triangle is $\frac{25}{2}$ and that the two legs are equal, so we can easily tell that the length of the two legs is $5$. Thus, the hypotenuse $AB = 5\sqrt{2}$ and $\angle{CAB} = \angle{ABC} = 45^{\circ}.$ Let's quickly define $F$ as the point that bisects $\angle{ACB}$ and $\overline{AB}$. Then, we can say that the area of the desired triangle is $DF \cdot AF$. Let $\overline{AD} = \overline{BE} = n.$ Since $D$ is one of the trisecting points of $\angle{ACB}, \angle{ACD} = 30^{\circ}.$ Because \[\angle{ADC} = 180^{\circ}-\angle{ACD}-\angle{CAB},\] \[\angle{ADC} = 180^{\circ} - 45^{\circ} - 30^{\circ} = 105^{\circ}.\] Now, we can employ the Law of Sines. It tells us that $\frac{\sin(\angle{ADC})}{5} = \frac{\sin(\angle{ACD})}{n}$. Plugging in our angle values, we get that \[\frac{\sin(105^{\circ})}{5} = \frac{\sin(30^{\circ})}{n}.\] It's easy to find that $\sin(105^{\circ}) = \sin(75^{\circ}) = \frac{\sqrt{2}+\sqrt{6}}{4},$ and that $\sin(30^{\circ}) = \frac{1}{2}$. Plugging in these values into our previous equation, we get \[\frac{\sqrt{2}+\sqrt{6}}{20} = \frac{1}{2n}.\] Cross multiplying gets us \[2n\sqrt{2}+2n\sqrt{6} = 20,\] and then we simplify like so: \[2n(\sqrt{2}+\sqrt{6}) = 20\rightarrow\] \[n(\sqrt{2}+\sqrt{6}) = 10\rightarrow\] \[n = \frac{10}{\sqrt{2}+\sqrt{6}}.\] Now, using our definition of $n$, we know that $DF$ = $\frac{AB}{2} - n = \frac{5\sqrt{2}}{2} - \frac{10}{\sqrt{2}+\sqrt{6}}$. We want to put this under one common denominator, which is pretty simple to execute. That leaves us with \[\frac{5\sqrt{2} \cdot \sqrt{2}+5\sqrt{2} \cdot \sqrt{6} - 20}{2\sqrt{2} + 2\sqrt{6}}=\] \[\frac{10+10\sqrt{3} - 20}{2\sqrt{2} + 2\sqrt{6}}=\] \[\frac{10\sqrt{3} - 10}{2(\sqrt{2} + \sqrt{6})}=\] \[\frac{2(5\sqrt{3} - 5)}{2(\sqrt{2} + \sqrt{6})}=\] \[\frac{5\sqrt{3} - 5}{\sqrt{2} + \sqrt{6}}.\] Whew. That was longer than expected. Anyways, quick inspection tells us that $AF = \frac{5\sqrt{2}}{2},$ so now we just have to do some simplifying to find the desired, $[CDE]$. Let's do that now. \[[CDE] = \frac{5\sqrt{3} - 5}{\sqrt{2} + \sqrt{6}} \cdot \frac{5\sqrt{2}}{2}=\] \[[CDE] = \frac{25\sqrt{6} - 25\sqrt{2}}{2(\sqrt{2} + \sqrt{6})}=\] \[[CDE] = \frac{25(\sqrt{2} - \sqrt{6})}{2(\sqrt{2} + \sqrt{6})}=\] (We need to take a quick conjugation break. Note that $(\sqrt{2} - \sqrt{6})^2 = 8 - 4\sqrt{3}.$) \[[CDE] = \frac{25(\sqrt{2} - \sqrt{6})}{2(\sqrt{2} + \sqrt{6})} \cdot \frac{(\sqrt{2} - \sqrt{6})}{(\sqrt{2} - \sqrt{6})}=\] \[[CDE] = \frac{200 - 100\sqrt{3}}{2(4)}=\] \[[CDE] =\boxed{\frac{50 - 25\sqrt{3}}{2} \text{(D)}.}\] ~Nickelslordm


Video Solution:

https://www.youtube.com/watch?v=JWMIsCS0Ksk

See Also

2015 AMC 10A (Problems • Answer Key • Resources)
Preceded by
Problem 18 		Followed by
Problem 20
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 10 Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2015_AMC_10A_Problems/Problem_19&oldid=219311"