Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2023 AMC 12B Problems/Problem 20"

m (→‎Solution 3(coord bash))
(→‎Solution 5 - Complex Number and Double Angle Formula)
 
(5 intermediate revisions by 3 users not shown)
Line 32: Line 32:
  
 
==Solution 3(coord bash)==
 
==Solution 3(coord bash)==
−
Let the orgin be the starting point of frog. Then, WLOG assume that after the first jump, it is at the point (2,0). Then, the range of all possible places the frog can jump to at its second jump is the circle with equation <math>(x-2)^2+y^2=2^2</math>.If it landed <math>1</math> unit within its starting point (the orgin), then it is inside the circle <math>x^2+y^2=1</math>. We clearly want the intersection point. So we're trying to solve the system of equations <math>x^2+y^2=1</math> and <math>(x-2)^2+y^2=2^2</math>. We have <math>x=\frac{1}{4}</math>, so <math>y=\pm\frac{\sqrt{15}}{4}</math>. Therefore, our final answer would be <math>\frac{\arcsin{\frac{\sqrt{15}}{8}}}{\pi}</math> (the angle we want divided by <math>2\pi</math>). But that is not one of our answer choices! Don't worry though, because
+
Let the orgin be the starting point of frog. Then, WLOG assume that after the first jump, it is at the point (2,0). Then, the range of all possible places the frog can jump to at its second jump is the circle with equation <math>(x-2)^2+y^2=2^2</math>.If it landed <math>1</math> unit within its starting point (the orgin), then it is inside the circle <math>x^2+y^2=1</math>. We clearly want the intersection point. So we're trying to solve the system of equations <math>x^2+y^2=1</math> and <math>(x-2)^2+y^2=2^2</math>. We have <math>x=\frac{1}{4}</math>, so <math>y=\pm\frac{\sqrt{15}}{4}</math>. Therefore, our desired answer would be <math>\frac{\arcsin{\frac{\sqrt{15}}{8}}}{\pi}</math> (the angle we want divided by <math>2\pi</math>). Since
  
−
<math>\arcsin{\frac{\sqrt{15}}{8}}=\arccos{\frac{7}{8}}=\arccos{(1-2 \cdot (\frac{1}{4})^{2})}=2\arcsin{\frac{1}{4}}</math>
+
<cmath>\arcsin{\frac{\sqrt{15}}{8}}=\arccos{\frac{7}{8}}=\arccos{(1-2 \cdot (\frac{1}{4})^{2})}=2\arcsin{\frac{1}{4}}</cmath>
  
−
where the last step holds by the double angle formula. By now, it is clear that our answer is <math>\boxed{(E)\frac{2\arcsin{\frac{1}{4}}}{\pi}}</math>.
+
where the last step holds by the double angle formula, our answer is <math>\boxed{(E)\frac{2\arcsin{\frac{1}{4}}}{\pi}}</math>.
 
~[[Ddk001]]
 
~[[Ddk001]]
  
 
==Solution 4 - Law of Cosines and Double Angle Formula==
 
==Solution 4 - Law of Cosines and Double Angle Formula==
−
Let A be Cyrus's starting point, B be the first point he jumps to (AB=2), and C be the second point he jumps to (BC=2). Let angle ABC be k, such that AC=1. The probability of AC<1 would therefore be 2k/360 (since C could be on either side of AB so there's two possible areas of having AC<1) = k/180 and converting to radians gives us k/pi. To find k we use the law of cosines.
+
Let <math>A</math> be Cyrus's starting point, <math>B</math> be the first point he jumps to (<math>AB = 2</math>), and <math>C</math> be the second point he jumps to (<math>BC = 2</math>). Let angle <math>ABC</math> be <math>k</math>, such that <math>AC = 1</math>. The probability of <math>AC < 1</math> would therefore be <math>\frac{2k}{360}</math> (since <math>C</math> could be on either side of <math>AB</math> so there are two possible areas of having <math>AC < 1</math>) which simplifies to <math>\frac{k}{180}</math>. Converting to radians gives us <math>\frac{k}{\pi}</math>. To find <math>k</math>, we use the law of cosines.
  
−
AC^2=AB^2+BC^2-2(AB)(BC)cos k
+
<cmath>AC^2 = AB^2 + BC^2 - 2 \cdot AB \cdot BC \cdot \cos k</cmath> <cmath>1^2 = 2^2 + 2^2 - 2 \cdot 2 \cdot 2 \cdot \cos k</cmath> <cmath>1 = 4 + 4 - 8 \cos k</cmath> <cmath>8 \cos k = 7</cmath> <cmath>\cos k = \frac{7}{8}</cmath> <cmath>k = \arccos\left(\frac{7}{8}\right) = \arcsin\left(\sqrt{1 - \left(\frac{7}{8}\right)^2}\right) = \arcsin\left(\sqrt{\frac{15}{64}}\right) = \arcsin\left(\frac{1}{4} \sqrt{\frac{15}{4}}\right) = \arcsin\left(\frac{1}{4} \sqrt{1 - \left(\frac{1}{4}\right)^2}\right)</cmath> <cmath>= \arcsin\left(\sin\left(\arcsin\left(\frac{1}{4}\right)\right) \cos\left(\arcsin\left(\frac{1}{4}\right)\right)\right) = \arcsin\left(\sin\left(2 \arcsin\left(\frac{1}{4}\right)\right)\right) = 2 \arcsin\left(\frac{1}{4}\right)</cmath>
  
−
1^2=2^2+2^2-2(2)(2)cos k
+
The probability is <cmath>\frac{k}{\pi} = \frac{2 \arcsin\left(\frac{1}{4}\right)}{\pi}</cmath>
  
−
1=4+4-8cos k
+
which is <math>E</math>.
  
−
8cos k=7
+
==Solution 5 - Complex Number and Double Angle Formula==
  
−
cos k=7/8
+
[[Image:2023_amc_12_A_p20.png|thumb|center|400px| ]]
  
−
k=arccos(7/8)=arcsin√(1-(7/8)^2)=arcsin(√15/64)=arcsin((1/4)(√15/4))=arcsin((1/4)(√(1-(1^4)^2)))=arcsin(sin(arcsin(1/4))cos(arcsin(1/4)))=arcsin(sin(2arcsin(1/4)))=2arcsin(1/4)
+
The frog jumps 2 units in one direction, which we can represent as <math> z_1 = 2e^{ia} </math>, where <math> a </math> is the angle in radians. Then, the frog jumps another 2 units in a different direction, represented as <math> z_2 = 2e^{ib} </math>, where <math> b </math> is the angle in radians.
  
−
The probability is k/pi=(2arcsin(1/4))/pi which is E.
+
The total displacement from the starting position is <math> z_3 = z_1 + z_2 = 2e^{ia} + 2e^{ib} </math>.
 +
 
 +
 
 +
We need to find the condition under which <math> |z_3| <= 1 </math>. This translates to:
 +
<cmath> |2e^{ia} + 2e^{ib}| <= 1 </cmath>
 +
 
 +
<cmath> |e^{ia} + e^{ib}| <= \frac{1}{2} </cmath>
 +
 
 +
<cmath> e^{ia} + e^{ib} = \cos(a) + i\sin(a) + \cos(b) + i\sin(b) </cmath>
 +
<cmath> = (\cos(a) + \cos(b)) + i(\sin(a) + \sin(b)) </cmath>
 +
 
 +
The magnitude squared of this sum is:
 +
<cmath> |e^{ia} + e^{ib}|^2 = (\cos(a) + \cos(b))^2 + (\sin(a) + \sin(b))^2 </cmath>
 +
<cmath> = \cos^2(a) + 2\cos(a)\cos(b) + \cos^2(b) + \sin^2(a) + 2\sin(a)\sin(b) + \sin^2(b) </cmath>
 +
<cmath> = 2 + 2(\cos(a)\cos(b) + \sin(a)\sin(b)) </cmath>
 +
<cmath> = 2 + 2\cos(a - b) </cmath>
 +
 
 +
Thus:
 +
<cmath> 2 + 2\cos(a - b) <= \frac{1}{4} </cmath>
 +
<cmath> 2\cos(a - b) <= -\frac{7}{4} </cmath>
 +
<cmath> \cos(a - b) <= -\frac{7}{8} </cmath>
 +
 
 +
Therefore, our desired answer would be <math>\frac{\arccos{\frac{7}{8}}}{\pi}</math> (the angle we want divided by <math>2\pi</math>). Since
 +
 
 +
<cmath> \arccos{\frac{7}{8}}=\arccos{(1-2 \cdot (\frac{1}{4})^{2})}=2\arcsin{\frac{1}{4}}</cmath>
 +
 
 +
where the last step holds by the double angle formula, our answer is <math>\boxed{(E)\frac{2\arcsin{\frac{1}{4}}}{\pi}}</math>.
 +
 
 +
~[https://artofproblemsolving.com/wiki/index.php/User:Cyantist luckuso]
  
 
==Video Solution 1 by OmegaLearn==
 
==Video Solution 1 by OmegaLearn==

Latest revision as of 23:57, 2 August 2024

Problem

Cyrus the frog jumps $2$ units in a direction, then $2$ more in another direction. What is the probability that he lands less than $1$ unit away from his starting position?

$\textbf{(A)}~\frac{1}{6}\qquad\textbf{(B)}~\frac{1}{5}\qquad\textbf{(C)}~\frac{\sqrt{3}}{8}\qquad\textbf{(D)}~\frac{\arctan \frac{1}{2}}{\pi}\qquad\textbf{(E)}~\frac{2\arcsin \frac{1}{4}}{\pi}$

Solution 1

2023AMC12BP20.png

Let Cyrus's starting position be $S$. WLOG, let the place Cyrus lands at for his first jump be $O$. From $O$, Cyrus can reach all the points on $\odot O$. The probability that Cyrus will land less than $1$ unit away from $S$ is $\frac{4 \alpha }{ 2 \pi}$.

\[\sin \alpha = \frac{ \frac12 }{2} = \frac14, \quad \alpha = \arcsin \frac14\]

Therefore, the answer is

\[\frac{4 \arcsin \frac14 }{ 2 \pi} = \boxed{\textbf{(E) } \frac{2 \arcsin \frac{1}{4}}{\pi}}\]

~isabelchen

Solution 2

Denote by $A_i$ the position after the $i$th jump. Thus, to fall into the region centered at $A_0$ and with radius 1, $\angle A_2 A_1 A_0 < 2 \arcsin \frac{1/2}{2} = 2 \arcsin \frac{1}{4}$.

Therefore, the probability is \[ \frac{2 \cdot 2 \arcsin \frac{1}{4}}{2 \pi} = \boxed{\textbf{(E) } \frac{2 \arcsin \frac{1}{4}}{\pi}}. \]

~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)

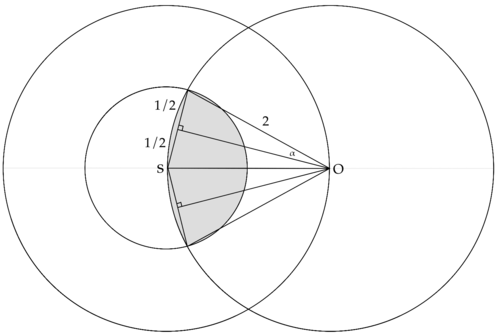
Solution 3(coord bash)

Let the orgin be the starting point of frog. Then, WLOG assume that after the first jump, it is at the point (2,0). Then, the range of all possible places the frog can jump to at its second jump is the circle with equation $(x-2)^2+y^2=2^2$.If it landed $1$ unit within its starting point (the orgin), then it is inside the circle $x^2+y^2=1$. We clearly want the intersection point. So we're trying to solve the system of equations $x^2+y^2=1$ and $(x-2)^2+y^2=2^2$. We have $x=\frac{1}{4}$, so $y=\pm\frac{\sqrt{15}}{4}$. Therefore, our desired answer would be $\frac{\arcsin{\frac{\sqrt{15}}{8}}}{\pi}$ (the angle we want divided by $2\pi$). Since

\[\arcsin{\frac{\sqrt{15}}{8}}=\arccos{\frac{7}{8}}=\arccos{(1-2 \cdot (\frac{1}{4})^{2})}=2\arcsin{\frac{1}{4}}\]

where the last step holds by the double angle formula, our answer is $\boxed{(E)\frac{2\arcsin{\frac{1}{4}}}{\pi}}$. ~Ddk001

Solution 4 - Law of Cosines and Double Angle Formula

Let $A$ be Cyrus's starting point, $B$ be the first point he jumps to ($AB = 2$), and $C$ be the second point he jumps to ($BC = 2$). Let angle $ABC$ be $k$, such that $AC = 1$. The probability of $AC < 1$ would therefore be $\frac{2k}{360}$ (since $C$ could be on either side of $AB$ so there are two possible areas of having $AC < 1$) which simplifies to $\frac{k}{180}$. Converting to radians gives us $\frac{k}{\pi}$. To find $k$, we use the law of cosines.

\[AC^2 = AB^2 + BC^2 - 2 \cdot AB \cdot BC \cdot \cos k\] \[1^2 = 2^2 + 2^2 - 2 \cdot 2 \cdot 2 \cdot \cos k\] \[1 = 4 + 4 - 8 \cos k\] \[8 \cos k = 7\] \[\cos k = \frac{7}{8}\] \[k = \arccos\left(\frac{7}{8}\right) = \arcsin\left(\sqrt{1 - \left(\frac{7}{8}\right)^2}\right) = \arcsin\left(\sqrt{\frac{15}{64}}\right) = \arcsin\left(\frac{1}{4} \sqrt{\frac{15}{4}}\right) = \arcsin\left(\frac{1}{4} \sqrt{1 - \left(\frac{1}{4}\right)^2}\right)\] \[= \arcsin\left(\sin\left(\arcsin\left(\frac{1}{4}\right)\right) \cos\left(\arcsin\left(\frac{1}{4}\right)\right)\right) = \arcsin\left(\sin\left(2 \arcsin\left(\frac{1}{4}\right)\right)\right) = 2 \arcsin\left(\frac{1}{4}\right)\]

The probability is \[\frac{k}{\pi} = \frac{2 \arcsin\left(\frac{1}{4}\right)}{\pi}\]

which is $E$.

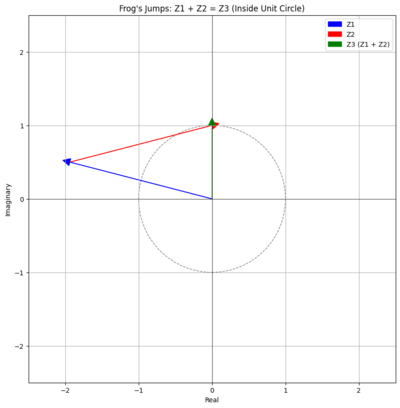
Solution 5 - Complex Number and Double Angle Formula

2023 amc 12 A p20.png

The frog jumps 2 units in one direction, which we can represent as $z_1 = 2e^{ia}$, where $a$ is the angle in radians. Then, the frog jumps another 2 units in a different direction, represented as $z_2 = 2e^{ib}$, where $b$ is the angle in radians.

The total displacement from the starting position is $z_3 = z_1 + z_2 = 2e^{ia} + 2e^{ib}$.


We need to find the condition under which $|z_3| <= 1$. This translates to: \[|2e^{ia} + 2e^{ib}| <= 1\]

\[|e^{ia} + e^{ib}| <= \frac{1}{2}\]

\[e^{ia} + e^{ib} = \cos(a) + i\sin(a) + \cos(b) + i\sin(b)\] \[= (\cos(a) + \cos(b)) + i(\sin(a) + \sin(b))\]

The magnitude squared of this sum is: \[|e^{ia} + e^{ib}|^2 = (\cos(a) + \cos(b))^2 + (\sin(a) + \sin(b))^2\] \[= \cos^2(a) + 2\cos(a)\cos(b) + \cos^2(b) + \sin^2(a) + 2\sin(a)\sin(b) + \sin^2(b)\] \[= 2 + 2(\cos(a)\cos(b) + \sin(a)\sin(b))\] \[= 2 + 2\cos(a - b)\]

Thus: \[2 + 2\cos(a - b) <= \frac{1}{4}\] \[2\cos(a - b) <= -\frac{7}{4}\] \[\cos(a - b) <= -\frac{7}{8}\]

Therefore, our desired answer would be $\frac{\arccos{\frac{7}{8}}}{\pi}$ (the angle we want divided by $2\pi$). Since

\[\arccos{\frac{7}{8}}=\arccos{(1-2 \cdot (\frac{1}{4})^{2})}=2\arcsin{\frac{1}{4}}\]

where the last step holds by the double angle formula, our answer is $\boxed{(E)\frac{2\arcsin{\frac{1}{4}}}{\pi}}$.

~luckuso

Video Solution 1 by OmegaLearn

https://youtu.be/nGZ9goJmg4Q

Video Solution

https://youtu.be/_i_d-C1cjyI

~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)

See Also

2023 AMC 12B (Problems • Answer Key • Resources)
Preceded by
Problem 19 		Followed by
Problem 21
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2023_AMC_12B_Problems/Problem_20&oldid=224335"