Difference between revisions of "2024 AMC 10A Problems/Problem 25"
(→Solution 2) |
(→Solution 3 (observation)) |
||
| (35 intermediate revisions by 21 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | {{duplicate|[[2024 AMC 10A Problems/Problem 25|2024 AMC 10A #25]] and [[2024 AMC 12A Problems/Problem 22|2024 AMC 12A #22]]}} | |
| − | <math>\ | + | ==Problem== |
| − | + | The figure below shows a dotted grid <math>8</math> cells wide and <math>3</math> cells tall consisting of <math>1''\times1''</math> squares. Carl places <math>1</math>-inch toothpicks along some of the sides of the squares to create a closed loop that does not intersect itself. The numbers in the cells indicate the number of sides of that square that are to be covered by toothpicks, and any number of toothpicks are allowed if no number is written. In how many ways can Carl place the toothpicks? | |
| + | <asy> | ||
| + | size(6cm); | ||
| + | for (int i=0; i<9; ++i) { | ||
| + | draw((i,0)--(i,3),dotted); | ||
| + | } | ||
| + | for (int i=0; i<4; ++i){ | ||
| + | draw((0,i)--(8,i),dotted); | ||
| + | } | ||
| + | for (int i=0; i<8; ++i) { | ||
| + | for (int j=0; j<3; ++j) { | ||
| + | if (j==1) { | ||
| + | label("1",(i+0.5,1.5)); | ||
| + | }}} | ||
| + | </asy> | ||
| + | <math>\textbf{(A) }130\qquad\textbf{(B) }144\qquad\textbf{(C) }146\qquad\textbf{(D) }162\qquad\textbf{(E) }196</math> | ||
| − | ==Solution 1== | + | ==(Best) Solution 1== |
| − | |||
| − | + | Observations: | |
| − | |||
| − | + | 1. You can not have a vertical line in any place other than the first and second columns and the last and second-to-last columns. | |
| − | |||
| − | |||
| − | + | 2. You can place a box around the top row or along the bottom row, otherwise all the solutions have a vertical line at the first and second columns and the last and second-to-last columns. | |
| − | |||
| − | + | Thus, Using casework, we can split this problem into 4 cases. However, we can focus on only the first one for right now. | |
| − | + | For case 1, we assume that the green lines shown below are given (always have toothpicks on them) | |
| − | + | [[Image:Scrasdfasd.png|600px]] | |
| − | + | [[Image:Screenshot 2024-11-08 192200.png|600px]] | |
| − | + | And the only toothpicks we can place that will connect to the red lines are to go horizontally inward: | |
| − | |||
| − | + | [[Image:Screenshot 2024-11-08 192516.png|600px]] | |
| − | + | Now, concentrate on the first row of squares. A toothpick can be placed on either the bottom or top and connected to a continuous squiggle by adding vertical toothpicks, for example, | |
| − | + | [[Image:Screenshot 2024-11-08 193401.png|600px]] | |
| − | + | How many squiggles are possible? | |
| − | + | [[Image:Screenshot 2024-11-08 194804.png|600px]] | |
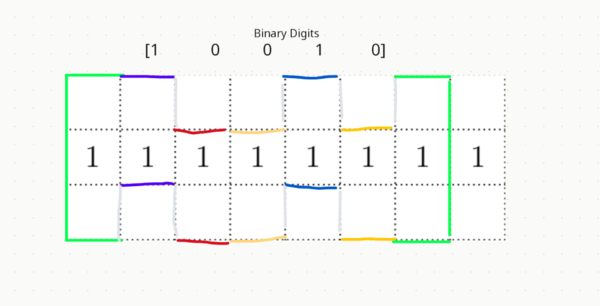
| − | + | We can summarize this by giving a high squiggle position a 1 and a low position a 0, thus we have a 6-digit binary sequence. Thus, we can have <math>2^6=64</math> ways to make this squiggle. | |
| − | + | Case 2: We can also, as the observations state, pull in one of the sides, thus we can have a squiggle with 5 binary digits. only utilizing the first 7 columns: | |
| − | |||
| − | + | [[Image:Screenshot 2024-11-08 195135.png|600px]] | |
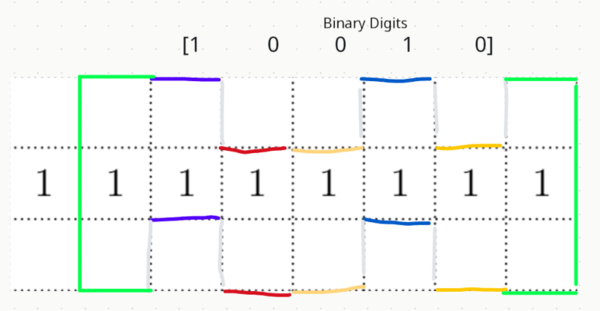
| − | + | Here, we only have 5 binary digits to work with, so there are <math>2^5=32</math> ways to make this squiggle. | |
| − | |||
| − | + | Case 3: Similarly, we can utilize the last 7 columns. | |
| − | + | [[Image:Screenshot 2024-11-08 195553.png|600px]] | |
| − | + | Again, we only have 5 binary digits to work with, so there are <math>2^5=32</math> ways to make this squiggle. | |
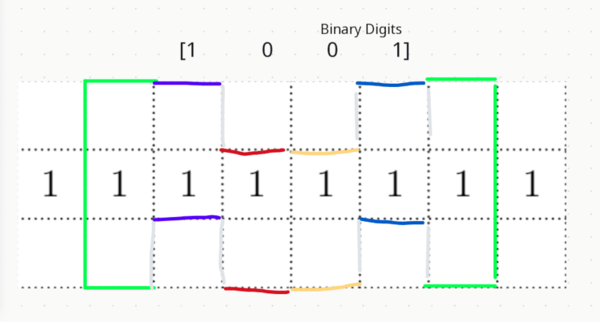
| − | + | Case 4: We can use an even smaller section. Using only the middle 6 columns gives us a 4-wide squiggle: | |
| + | |||
| + | [[Image:Screenshot 2024-11-08 195945.png|600px]] | ||
| + | |||
| + | Thus, there are <math>2^4=16</math> ways to make this squiggle. | ||
| + | |||
| + | Adding up all our cases: <math>64+32+32+16=144</math> | ||
| + | |||
| + | However, there are two more ways to draw a qualifying shape: | ||
| + | |||
| + | [[Image:Screenshot 2024-11-08 200416.png|600px]] | ||
| + | |||
| + | We can draw a rectangle like that in the first row or third row. Thus, we have a grand total of | ||
| + | <math>144+2=\boxed{\textbf{(C) }146}</math> ways. | ||
| + | |||
| + | |||
| + | A note to (potential) editors: | ||
| + | This answer was not made to be concise or especially professional. It was made to explicitly explain this problem in a way so that it is easy to understand and follow. | ||
| + | |||
| + | ~hermanboxcar5 | ||
| + | |||
| + | Notes: | ||
| + | Remember these are the ONLY possible cases. It is impossible to cross through the rows of boxes of ones to connect the loop around the bottom since then the loop would intersect itself if you are to put only one toothpick on each box with a one (a more clear definition of Observation 1). | ||
| + | You are not undercounting by only counting the binary digits on the top, because all of the digits on one side will have corresponding opposite digits on the other. | ||
| + | |||
| + | ~juwushu | ||
| + | |||
| + | ==Solution 2 (Cheese)== | ||
| + | Notice that for any case where the closed loop does not connect from the top side of the ones and bottom side of the ones, there are two of these cases. A cheese solution can be found from this; noting that B and C are the only two options to each other, and, being two apart, with people likely to forget this case, <math>\boxed{\textbf{(C) }146}</math> is likely to be the correct answer. | ||
| + | Cheese solution done by [[User:Juwushu|juwushu]]. | ||
| + | |||
| + | ==Solution 3 (Observation)== | ||
| + | |||
| + | We have <math>2</math> cases where the loop does not go through the middle. | ||
| + | |||
| + | If the loop goes through the middle, we must have a full column on <math>(0,8),(0,7),(1,8),(1,7).</math> Then we have <math>6,5,5,4</math> empty middle squares. For each one we can have one on top or one on bottom, so <math>2^6+2^5+2^5+2^4=144.</math> Notice that for each case of fixed toothpicks, there is only one way to form the loop. Then we just add <math>2+144=\boxed{\textbf{(C) } 146.}</math> | ||
| + | |||
| + | ~nevergonnagiveup | ||
| + | |||
| + | == Video Solution by Power Solve == | ||
| + | https://www.youtube.com/watch?v=FRNbJ5wIGRo | ||
| + | |||
| + | ==Video Solution By SpreadTheMathLove== | ||
| + | https://www.youtube.com/watch?v=huMQ9J7rIj0&t=77s | ||
| + | |||
| + | ==See also== | ||
| + | {{AMC10 box|year=2024|ab=A|num-b=24|after=Last Problem}} | ||
| + | {{AMC12 box|year=2024|ab=A|num-b=21|num-a=23}} | ||
| + | {{MAA Notice}} | ||
Latest revision as of 14:53, 20 November 2024
- The following problem is from both the 2024 AMC 10A #25 and 2024 AMC 12A #22, so both problems redirect to this page.
Contents
Problem
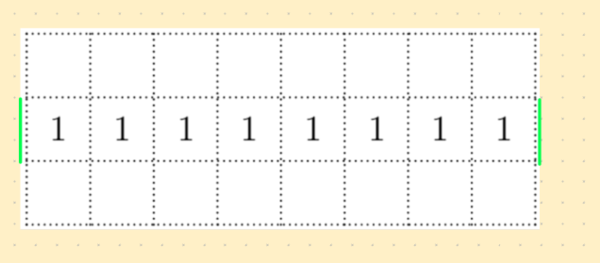
The figure below shows a dotted grid ![]() cells wide and
cells wide and ![]() cells tall consisting of
cells tall consisting of ![]() squares. Carl places
squares. Carl places ![]() -inch toothpicks along some of the sides of the squares to create a closed loop that does not intersect itself. The numbers in the cells indicate the number of sides of that square that are to be covered by toothpicks, and any number of toothpicks are allowed if no number is written. In how many ways can Carl place the toothpicks?
-inch toothpicks along some of the sides of the squares to create a closed loop that does not intersect itself. The numbers in the cells indicate the number of sides of that square that are to be covered by toothpicks, and any number of toothpicks are allowed if no number is written. In how many ways can Carl place the toothpicks?
![[asy] size(6cm); for (int i=0; i<9; ++i) { draw((i,0)--(i,3),dotted); } for (int i=0; i<4; ++i){ draw((0,i)--(8,i),dotted); } for (int i=0; i<8; ++i) { for (int j=0; j<3; ++j) { if (j==1) { label("1",(i+0.5,1.5)); }}} [/asy]](http://latex.artofproblemsolving.com/9/d/1/9d176dfd41e3e8e875c6b5d84fdb4f5e4f7d43a6.png)
![]()
(Best) Solution 1
Observations:
1. You can not have a vertical line in any place other than the first and second columns and the last and second-to-last columns.
2. You can place a box around the top row or along the bottom row, otherwise all the solutions have a vertical line at the first and second columns and the last and second-to-last columns.
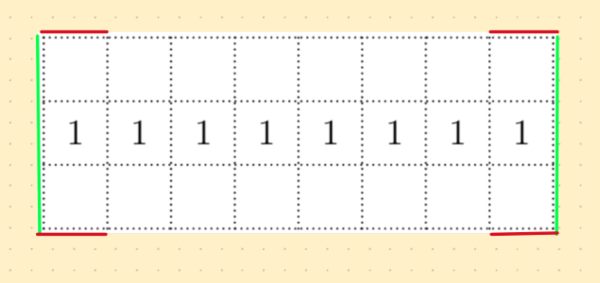
Thus, Using casework, we can split this problem into 4 cases. However, we can focus on only the first one for right now.
For case 1, we assume that the green lines shown below are given (always have toothpicks on them)
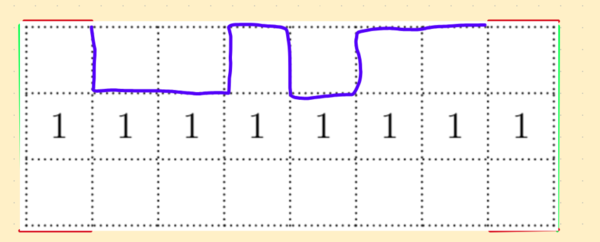
And the only toothpicks we can place that will connect to the red lines are to go horizontally inward:
Now, concentrate on the first row of squares. A toothpick can be placed on either the bottom or top and connected to a continuous squiggle by adding vertical toothpicks, for example,
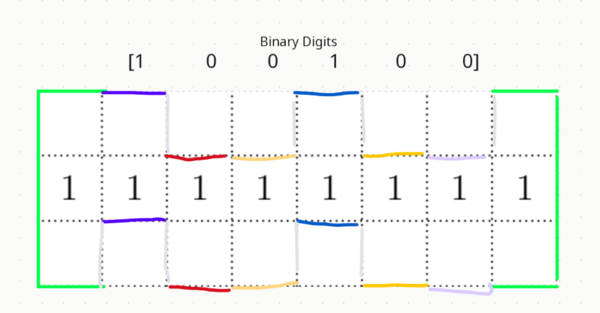
How many squiggles are possible?
We can summarize this by giving a high squiggle position a 1 and a low position a 0, thus we have a 6-digit binary sequence. Thus, we can have ![]() ways to make this squiggle.
ways to make this squiggle.
Case 2: We can also, as the observations state, pull in one of the sides, thus we can have a squiggle with 5 binary digits. only utilizing the first 7 columns:
Here, we only have 5 binary digits to work with, so there are ![]() ways to make this squiggle.
ways to make this squiggle.
Case 3: Similarly, we can utilize the last 7 columns.
Again, we only have 5 binary digits to work with, so there are ![]() ways to make this squiggle.
ways to make this squiggle.
Case 4: We can use an even smaller section. Using only the middle 6 columns gives us a 4-wide squiggle:
Thus, there are ![]() ways to make this squiggle.
ways to make this squiggle.
Adding up all our cases: ![]()
However, there are two more ways to draw a qualifying shape:
We can draw a rectangle like that in the first row or third row. Thus, we have a grand total of
![]() ways.
ways.
A note to (potential) editors:
This answer was not made to be concise or especially professional. It was made to explicitly explain this problem in a way so that it is easy to understand and follow.
~hermanboxcar5
Notes: Remember these are the ONLY possible cases. It is impossible to cross through the rows of boxes of ones to connect the loop around the bottom since then the loop would intersect itself if you are to put only one toothpick on each box with a one (a more clear definition of Observation 1). You are not undercounting by only counting the binary digits on the top, because all of the digits on one side will have corresponding opposite digits on the other.
~juwushu
Solution 2 (Cheese)
Notice that for any case where the closed loop does not connect from the top side of the ones and bottom side of the ones, there are two of these cases. A cheese solution can be found from this; noting that B and C are the only two options to each other, and, being two apart, with people likely to forget this case, ![]() is likely to be the correct answer.
Cheese solution done by juwushu.
is likely to be the correct answer.
Cheese solution done by juwushu.
Solution 3 (Observation)
We have ![]() cases where the loop does not go through the middle.
cases where the loop does not go through the middle.
If the loop goes through the middle, we must have a full column on ![]() Then we have
Then we have ![]() empty middle squares. For each one we can have one on top or one on bottom, so
empty middle squares. For each one we can have one on top or one on bottom, so ![]() Notice that for each case of fixed toothpicks, there is only one way to form the loop. Then we just add
Notice that for each case of fixed toothpicks, there is only one way to form the loop. Then we just add ![]()
~nevergonnagiveup
Video Solution by Power Solve
https://www.youtube.com/watch?v=FRNbJ5wIGRo
Video Solution By SpreadTheMathLove
https://www.youtube.com/watch?v=huMQ9J7rIj0&t=77s
See also
| 2024 AMC 10A (Problems • Answer Key • Resources) | ||
| Preceded by Problem 24 |
Followed by Last Problem | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
| 2024 AMC 12A (Problems • Answer Key • Resources) | |
| Preceded by Problem 21 |
Followed by Problem 23 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.