Difference between revisions of "Unit circle"
| Line 16: | Line 16: | ||
We can also prove one of he fundamental theorems of [[trigonometry]]: <math>\sin^2{\theta}+\cos^2{\theta}=1</math>. The proof is as follows: | We can also prove one of he fundamental theorems of [[trigonometry]]: <math>\sin^2{\theta}+\cos^2{\theta}=1</math>. The proof is as follows: | ||
| + | |||
We see that the length and width of the triangle in the diagram above left are <math>\sin{\theta}</math> and <math>\cos{\theta}</math>, respectively. We use the [[pythagorean theorem]] to get: | We see that the length and width of the triangle in the diagram above left are <math>\sin{\theta}</math> and <math>\cos{\theta}</math>, respectively. We use the [[pythagorean theorem]] to get: | ||
Revision as of 19:48, 13 October 2007
| This is an AoPSWiki Word of the Week for Oct 11-17 |
A unit circle is a circle whose radius has length 1.
In the Cartesian coordinate system, an equation of the form 
defines a unit circle with center 
.
Trigonometry
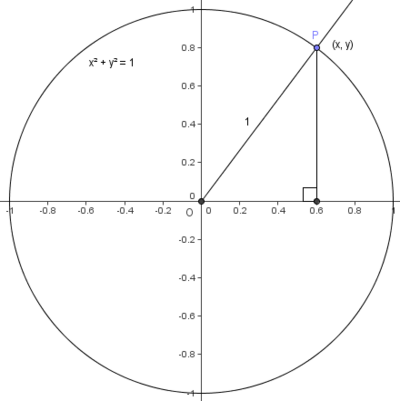
An unit circle centered at the origin can be used to calculate values for the basic trigonometric functions. Suppose we draw a ray starting from the origin and meeting the positive x-axis with an angle of 
. If we drop a perpendicular from the point of intersection between the ray and the circle, we have a right triangle with hypotenuse of 
.
Using the definitions 
and 
, we find that 
and 
.
We can read off values for sine and cosine of an angle this way; we can draw the angle and approximate the x and y coordinates of the intersection.
We can also prove one of he fundamental theorems of trigonometry: 
. The proof is as follows:
We see that the length and width of the triangle in the diagram above left are 
and 
, respectively. We use the pythagorean theorem to get:
This article is a stub. Help us out by expanding it.
