Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2005 AMC 12A Problems/Problem 16"

(→‎See also)
(→‎Solution)
Line 25: Line 25:
  
 
== Solution ==
 
== Solution ==
 +
 +
===Solution 1===
 
[[Image:2005_12A_AMC-16b.png]]
 
[[Image:2005_12A_AMC-16b.png]]
  
Line 32: Line 34:
  
 
Quite obviously <math>r > s = 1</math>, so <math>r = 9</math> and <math>\frac rs = \frac 91 = 9 \Longrightarrow \mathrm{(D)}</math>.
 
Quite obviously <math>r > s = 1</math>, so <math>r = 9</math> and <math>\frac rs = \frac 91 = 9 \Longrightarrow \mathrm{(D)}</math>.
 +
 +
===Solution 2===
 +
 +
Don't do this unless really really desperate. But I actually solved this with a ruler (try and see!!). Let <math>s=1</math> and find <math>r</math> in terms of <math>s</math>. The rest is easy.
 +
 +
Solution by franzliszt
  
 
== See also ==
 
== See also ==

Revision as of 19:07, 11 July 2020

Problem

Three circles of radius $s$ are drawn in the first quadrant of the $xy$-plane. The first circle is tangent to both axes, the second is tangent to the first circle and the $x$-axis, and the third is tangent to the first circle and the $y$-axis. A circle of radius $r > s$ is tangent to both axes and to the second and third circles. What is $r/s$?

[asy] unitsize(3mm); defaultpen(linewidth(.8pt)+fontsize(10pt)); dotfactor=3; pair O0=(9,9), O1=(1,1), O2=(3,1), O3=(1,3); pair P0=O0+9*dir(-45), P3=O3+dir(70); pair[] ps={O0,O1,O2,O3}; dot(ps); draw(Circle(O0,9)); draw(Circle(O1,1)); draw(Circle(O2,1)); draw(Circle(O3,1)); draw(O0--P0,linetype("3 3")); draw(O3--P3,linetype("2 2")); draw((0,0)--(18,0)); draw((0,0)--(0,18)); label("$r$",midpoint(O0--P0),NE); label("$s$",(-1.5,4)); draw((-1,4)--midpoint(O3--P3));[/asy]

$(\mathrm {A}) \ 5 \qquad (\mathrm {B}) \ 6 \qquad (\mathrm {C})\ 8 \qquad (\mathrm {D}) \ 9 \qquad (\mathrm {E})\ 10$

Solution

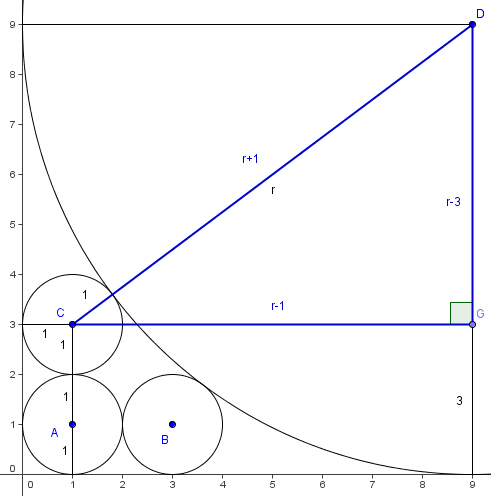
Solution 1

2005 12A AMC-16b.png

Without loss of generality, let $s = 1$. Draw the segment between the center of the third circle and the large circle; this has length $r+1$. We then draw the radius of the large circle that is perpendicular to the x-axis, and draw the perpendicular from this radius to the center of the third circle. This gives us a right triangle with legs $r-3,r-1$ and hypotenuse $r+1$. The Pythagorean Theorem yields:

$(r-3)^2 + (r-1)^2 = (r+1)^2$
$r^2 - 10r + 9 = 0$
$r = 1, 9$

Quite obviously $r > s = 1$, so $r = 9$ and $\frac rs = \frac 91 = 9 \Longrightarrow \mathrm{(D)}$.

Solution 2

Don't do this unless really really desperate. But I actually solved this with a ruler (try and see!!). Let $s=1$ and find $r$ in terms of $s$. The rest is easy.

Solution by franzliszt

See also

2005 AMC 12A (Problems • Answer Key • Resources)
Preceded by
Problem 15 		Followed by
Problem 17
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2005_AMC_12A_Problems/Problem_16&oldid=128109"