Difference between revisions of "2002 AMC 12B Problems/Problem 18"
(→Solution 2) |
(→Solution 2) |
||
| Line 15: | Line 15: | ||
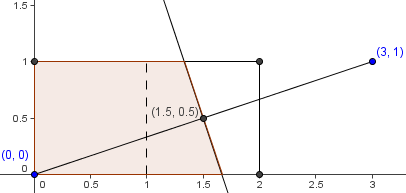
The region containing the points closer to <math>(0,0)</math> than to <math>(3,1)</math> is bounded by the [[perpendicular bisector]] of the segment with endpoints <math>(0,0),(3,1)</math>. The perpendicular bisector passes through midpoint of <math>(0,0),(3,1)</math>, which is <math>\left(\frac 32, \frac 12\right)</math>, the center of the [[unit square]] with coordinates <math>(1,0),(2,0),(2,1),(1,1)</math>. Thus, it cuts the unit square into two equal halves of area <math>1/2</math>. The total area of the rectangle is <math>2</math>, so the area closer to the origin than to <math>(3,1)</math> and in the rectangle is <math>2 - \frac 12 = \frac 32</math>. The probability is <math>\frac{3/2}{2} = \frac 34 \Rightarrow \mathrm{(C)}</math>. | The region containing the points closer to <math>(0,0)</math> than to <math>(3,1)</math> is bounded by the [[perpendicular bisector]] of the segment with endpoints <math>(0,0),(3,1)</math>. The perpendicular bisector passes through midpoint of <math>(0,0),(3,1)</math>, which is <math>\left(\frac 32, \frac 12\right)</math>, the center of the [[unit square]] with coordinates <math>(1,0),(2,0),(2,1),(1,1)</math>. Thus, it cuts the unit square into two equal halves of area <math>1/2</math>. The total area of the rectangle is <math>2</math>, so the area closer to the origin than to <math>(3,1)</math> and in the rectangle is <math>2 - \frac 12 = \frac 32</math>. The probability is <math>\frac{3/2}{2} = \frac 34 \Rightarrow \mathrm{(C)}</math>. | ||
| + | |||
=== Solution 2 === | === Solution 2 === | ||
Revision as of 19:16, 20 August 2019
Contents
[hide]Problem
A point ![]() is randomly selected from the rectangular region with vertices
is randomly selected from the rectangular region with vertices ![]() . What is the probability that
. What is the probability that ![]() is closer to the origin than it is to the point
is closer to the origin than it is to the point ![]() ?
?
![]()
Solution
Solution 1
The region containing the points closer to ![]() than to
than to ![]() is bounded by the perpendicular bisector of the segment with endpoints
is bounded by the perpendicular bisector of the segment with endpoints ![]() . The perpendicular bisector passes through midpoint of
. The perpendicular bisector passes through midpoint of ![]() , which is
, which is ![]() , the center of the unit square with coordinates
, the center of the unit square with coordinates ![]() . Thus, it cuts the unit square into two equal halves of area
. Thus, it cuts the unit square into two equal halves of area ![]() . The total area of the rectangle is
. The total area of the rectangle is ![]() , so the area closer to the origin than to
, so the area closer to the origin than to ![]() and in the rectangle is
and in the rectangle is ![]() . The probability is
. The probability is ![]() .
.
Solution 2
Assume that the point ![]() is randomly chosen within the rectangle with vertices
is randomly chosen within the rectangle with vertices ![]() ,
, ![]() ,
, ![]() ,
, ![]() . In this case, the region for
. In this case, the region for ![]() to be closer to the origin than to point
to be closer to the origin than to point ![]() occupies exactly
occupies exactly ![]() of the area of the rectangle, or
of the area of the rectangle, or ![]() square units.
square units.
If ![]() is chosen within the square with vertices
is chosen within the square with vertices ![]() ,
, ![]() ,
, ![]() ,
, ![]() which has area
which has area ![]() square unit, it is for sure closer to
square unit, it is for sure closer to ![]() .
.
Now if ![]() can only be chosen within the rectangle with vertices
can only be chosen within the rectangle with vertices ![]() ,
, ![]() ,
, ![]() ,
, ![]() , then the square region is removed and the area for
, then the square region is removed and the area for ![]() to be closer to
to be closer to ![]() is then decreased by
is then decreased by ![]() square unit, left with only
square unit, left with only ![]() square unit.
square unit.
Thus the probability that ![]() is closer to
is closer to ![]() is
is ![]() and that of
and that of ![]() is closer to the origin is
is closer to the origin is ![]() .
. ![]()
~ Nafer
See also
| 2002 AMC 12B (Problems • Answer Key • Resources) | |
| Preceded by Problem 17 |
Followed by Problem 19 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()