Difference between revisions of "2002 AIME I Problems/Problem 2"
m |
m (→See also) |
||
| Line 8: | Line 8: | ||
== See also == | == See also == | ||
| − | + | {{AIME box|year=2002|n=I|num-b=1|num-a=3}} | |
| − | |||
| − | |||
| − | |||
| − | |||
Revision as of 14:11, 25 November 2007
Problem
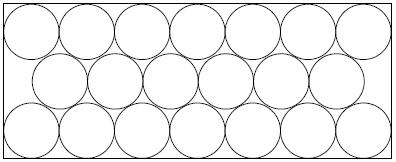
The diagram shows twenty congruent circles arranged in three rows and enclosed in a rectangle. The circles are tangent to one another and to the sides of the rectangle as shown in the diagram. The ratio of the longer dimension of the rectangle to the shorter dimension can be written as 
where 
and 
are positive integers. Find 
.
Solution
This problem needs a solution. If you have a solution for it, please help us out by adding it.
See also
| 2002 AIME I (Problems • Answer Key • Resources) | ||
| Preceded by Problem 1 |
Followed by Problem 3 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||
