Difference between revisions of "2023 AMC 12B Problems/Problem 17"
m (→Video Solution) |
|||
| Line 80: | Line 80: | ||
https://youtu.be/uVHCLHBWWJM | https://youtu.be/uVHCLHBWWJM | ||
| − | ==Video Solution== | + | ==Video Solution 2== |
https://youtu.be/3w59Hikefqs | https://youtu.be/3w59Hikefqs | ||
| Line 86: | Line 86: | ||
~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com) | ~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com) | ||
| − | |||
==See Also== | ==See Also== | ||
{{AMC12 box|year=2023|ab=B|num-b=16|num-a=18}} | {{AMC12 box|year=2023|ab=B|num-b=16|num-a=18}} | ||
{{MAA Notice}} | {{MAA Notice}} | ||
Latest revision as of 08:52, 4 December 2023
Contents
[hide]Problem
Triangle ![]() has side lengths in arithmetic progression, and the smallest side has length
has side lengths in arithmetic progression, and the smallest side has length ![]() If the triangle has an angle of
If the triangle has an angle of ![]() what is the area of
what is the area of ![]() ?
?
![]()
Solution 1
The length of the side opposite to the angle with ![]() is longest.
We denote its value as
is longest.
We denote its value as ![]() .
.
Because three side lengths form an arithmetic sequence, the middle-valued side length is ![]() .
.
Following from the law of cosines, we have
![]()
By solving this equation, we get ![]() .
Thus,
.
Thus, ![]() .
.
Therefore, the area of the triangle is
![]()
~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)
Solution 2
Let the side lengths of ![]() be
be ![]() ,
, ![]() , and
, and ![]() , where
, where ![]() . As
. As ![]() is the longest side, the angle opposite to it will be
is the longest side, the angle opposite to it will be ![]() .
.
By the law of Cosine
![]()
![]()
![]()
![]() As
As ![]() ,
, ![]() .
.
Therefore, ![]()
Solution 3
Let the side lengths of ![]() be
be ![]() ,
, ![]() , and
, and ![]() , where
, where ![]() . As
. As ![]() is the longest side, the angle opposite to it will be
is the longest side, the angle opposite to it will be ![]() .
.
By the law of Cosine
![]()
![]()
![]()
![]()
![]() As
As ![]() ,
, ![]() ,
, ![]()
Therefore, ![]()
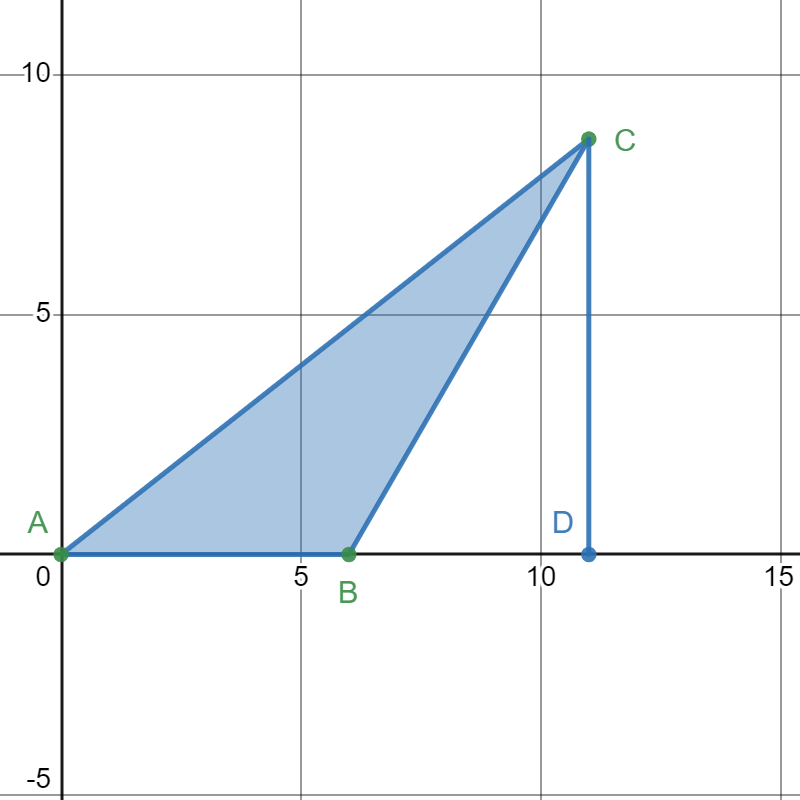
Solution 4 (Analytic Geometry)
Since the triangle's longest side must correspond to the ![]() angle, the triangle is unique. By analytic geometry, we construct the following plot.
angle, the triangle is unique. By analytic geometry, we construct the following plot.
We know the coordinates of point ![]() being the origin and
being the origin and ![]() being
being ![]() . Constructing the line which point
. Constructing the line which point ![]() can lay on, here since
can lay on, here since ![]() ,
, ![]() is on the line
is on the line ![]()
I denote ![]() as the perpendicular line from
as the perpendicular line from ![]() to
to ![]() , and assume
, and assume ![]() . Here we know
. Here we know ![]() is a
is a ![]() triangle. Hence
triangle. Hence ![]() and
and ![]() .
.
Furthermore, due to the arithmetic progression, we know ![]() . Hence, in
. Hence, in ![]() ,
, ![]()
![]()
Thus, the area is equal to ![]() .
.
~Prof. Joker
Video Solution 1 by OmegaLearn
Video Solution 2
~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)
See Also
| 2023 AMC 12B (Problems • Answer Key • Resources) | |
| Preceded by Problem 16 |
Followed by Problem 18 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.