2004 AMC 12B Problems/Problem 21
Problem
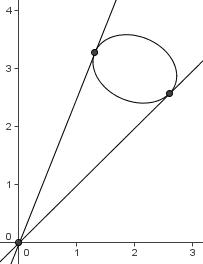
The graph of ![]() is an ellipse in the first quadrant of the
is an ellipse in the first quadrant of the ![]() -plane. Let
-plane. Let ![]() and
and ![]() be the maximum and minimum values of
be the maximum and minimum values of ![]() over all points
over all points ![]() on the ellipse. What is the value of
on the ellipse. What is the value of ![]() ?
?
![]()
Solution
![]() represents the slope of a line passing through the origin. It follows that since a line
represents the slope of a line passing through the origin. It follows that since a line ![]() intersects the ellipse at either
intersects the ellipse at either ![]() or
or ![]() points, the minimum and maximum are given when the line
points, the minimum and maximum are given when the line ![]() is a tangent, with only one point of intersection. Substituting,
is a tangent, with only one point of intersection. Substituting,
![]() Rearranging by the degree of
Rearranging by the degree of ![]() ,
,
![]() Since the line
Since the line ![]() , we want the discriminant,
, we want the discriminant,
![]() to be equal to
to be equal to ![]() . We want
. We want ![]() , which is the sum of the roots of the above quadratic. By Vieta’s formulas, that is
, which is the sum of the roots of the above quadratic. By Vieta’s formulas, that is ![]() .
.
See also
| 2004 AMC 12B (Problems • Answer Key • Resources) | |
| Preceded by Problem 20 |
Followed by Problem 22 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.