Difference between revisions of "2018 AIME II Problems/Problem 14"
(→Solution 4 (Projective geometry)) |
(→Solution 4 (Projective geometry)) |
||
| Line 71: | Line 71: | ||
==Solution 4 (Projective geometry)== | ==Solution 4 (Projective geometry)== | ||
| − | [[File:2018 AIME II 14.png| | + | [[File:2018 AIME II 14.png|500px|right]] |
<i><b>Lemma</b></i> | <i><b>Lemma</b></i> | ||
| Line 80: | Line 80: | ||
Let <math>E</math> be point of crossing <math>AX</math> and <math>BQ.</math> We make projective transformation such that circle <math>\omega</math> maps into the circle and point <math>E</math> maps into the center of new circle point <math>I.</math> We denote images using notification <math>X \rightarrow X'.</math> | Let <math>E</math> be point of crossing <math>AX</math> and <math>BQ.</math> We make projective transformation such that circle <math>\omega</math> maps into the circle and point <math>E</math> maps into the center of new circle point <math>I.</math> We denote images using notification <math>X \rightarrow X'.</math> | ||
| + | [[File:2018 AIME II 14a.png|300px|right]] | ||
| + | <math>BCQP</math> maps into <math>B'C'Q'P'</math>, so lines <math>B'Q'</math> and <math>A'X'</math> be the diameters. | ||
| + | This implies <math>P'Q'||B'C', \angle B'P'Q' = \angle B'C'Q' = 90^\circ \implies B'C'Q'P'</math> be a square. | ||
| − | <math> | + | Therefore <math>M'N'</math> be the diameter <math>\implies P'C', B'Q',</math> be diagonals of the square. <math>M'N'</math> and <math>X'Y'</math> be midlines which crossing in the center <math>I.</math> Therefore ines <math>PC, MN, AX,</math> and <math>BQ</math> are concurrent. |
| − | + | ||
| − | Therefore <math> | + | Lines <math>P'Q'||M'N' ||B'C' \implies PQ, MN</math> and <math>BC</math> are concurrent. |
| − | |||
| − | + | <i><b>Solution</b></i> | |
| + | The cross-ratio associated with a list of four collinear points <math>A,P,M,D</math> is defined as <cmath>(A,P;M,B)={\frac {AP\cdot MB}{AB\cdot PM}}.</cmath> | ||
| + | The cross-ratio be <i><b>projective invariant</b></i> of a quadruple of collinear points, so | ||
| + | <cmath>(A,P; M,B) = {\frac {A'P'\cdot M'B'}{A'B'\cdot P'M'}} = \frac {M'B'}{P'M'} = 1.</cmath> | ||
| + | <cmath>(A,P; M,B)={\frac {3\cdot (7 - AM)}{7\cdot (AM - 3)}} = 1 \implies AM = \frac {21}{5} \implies AN = AM = \frac {21}{5}.</cmath> | ||
| + | <cmath>(A,Q;N,C)={\frac {AQ\cdot NC}{AC\cdot QN}} = \frac {AQ\cdot (AC- AN)}{AC\cdot (AN-AQ)} = 1.</cmath> | ||
| + | <cmath>AQ \cdot (8 - \frac{21}{5}) = 8 \cdot (\frac{21}{5} – AQ) \implies AQ = \frac{168}{59}.</cmath> | ||
Revision as of 11:53, 12 June 2022
Contents
[hide]Problem
The incircle ![]() of triangle
of triangle ![]() is tangent to
is tangent to ![]() at
at ![]() . Let
. Let ![]() be the other intersection of
be the other intersection of ![]() with
with ![]() . Points
. Points ![]() and
and ![]() lie on
lie on ![]() and
and ![]() , respectively, so that
, respectively, so that ![]() is tangent to
is tangent to ![]() at
at ![]() . Assume that
. Assume that ![]() ,
, ![]() ,
, ![]() , and
, and ![]() , where
, where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]() .
.
Diagram
![[asy] size(200); import olympiad; defaultpen(linewidth(1)+fontsize(12)); pair A,B,C,P,Q,Wp,X,Y,Z; B=origin; C=(6.75,0); A=IP(CR(B,7),CR(C,8)); path c=incircle(A,B,C); Wp=IP(c,A--C); Z=IP(c,A--B); X=IP(c,B--C); Y=IP(c,A--X); pair I=incenter(A,B,C); P=extension(A,B,Y,Y+dir(90)*(Y-I)); Q=extension(A,C,P,Y); draw(A--B--C--cycle, black+1); draw(c^^A--X^^P--Q); pen p=4+black; dot("$A$",A,N,p); dot("$B$",B,SW,p); dot("$C$",C,SE,p); dot("$X$",X,S,p); dot("$Y$",Y,dir(55),p); dot("$W$",Wp,E,p); dot("$Z$",Z,W,p); dot("$P$",P,W,p); dot("$Q$",Q,E,p); MA("\beta",C,X,A,0.3,black); MA("\alpha",B,A,X,0.7,black); [/asy]](http://latex.artofproblemsolving.com/4/0/7/4073dcacb93163e2fca4cd2a02d09ff864a1f50b.png)
Solution 1
Let the sides ![]() and
and ![]() be tangent to
be tangent to ![]() at
at ![]() and
and ![]() , respectively. Let
, respectively. Let ![]() and
and ![]() . Because
. Because ![]() and
and ![]() are both tangent to
are both tangent to ![]() and
and ![]() and
and ![]() subtend the same arc of
subtend the same arc of ![]() , it follows that
, it follows that ![]() . By equal tangents,
. By equal tangents, ![]() . Applying the Law of Sines to
. Applying the Law of Sines to ![]() yields
yields ![]() Similarly, applying the Law of Sines to
Similarly, applying the Law of Sines to ![]() gives
gives ![]() It follows that
It follows that ![]() implying
implying ![]() . Applying the same argument to
. Applying the same argument to ![]() yields
yields ![]() from which
from which ![]() . The requested sum is
. The requested sum is ![]() .
.
Solution 2 (Projective)
Let the incircle of ![]() be tangent to
be tangent to ![]() and
and ![]() at
at ![]() and
and ![]() . By Brianchon's theorem on tangential hexagons
. By Brianchon's theorem on tangential hexagons ![]() and
and ![]() , we know that
, we know that ![]() and
and ![]() are concurrent at a point
are concurrent at a point ![]() . Let
. Let ![]() . Then by La Hire's
. Then by La Hire's ![]() lies on the polar of
lies on the polar of ![]() so
so ![]() lies on the polar of
lies on the polar of ![]() . Therefore,
. Therefore, ![]() also passes through
also passes through ![]() . Then projecting through
. Then projecting through ![]() , we have
, we have
![]() Therefore,
Therefore, ![]() . Since
. Since ![]() we know that
we know that ![]() and
and ![]() . Therefore,
. Therefore, ![]() and
and ![]() . Since
. Since ![]() , we also have
, we also have ![]() . Solving for
. Solving for ![]() , we obtain
, we obtain ![]() .
😃
-Vfire
.
😃
-Vfire
Solution 3 (Combination of Law of Sine and Law of Cosine)
Let the center of the incircle of ![]() be
be ![]() . Link
. Link ![]() and
and ![]() . Then we have
. Then we have ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Let the incircle of ![]() be tangent to
be tangent to ![]() and
and ![]() at
at ![]() and
and ![]() , let
, let ![]() and
and ![]() .
.
Use Law of Sine in ![]() and
and ![]() , we have
, we have
![]()
![]()
therefore we have
![]()
Solve this equation, we have ![]()
As a result, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]()
So, ![]()
Use Law of Cosine in ![]() and
and ![]() , we have
, we have
![]()

And we have
![]()
So

Solve this equation, we have ![]()
As a result, ![]()
So, the final answer of this question is ![]()
~Solution by ![]() (Frank FYC)
(Frank FYC)
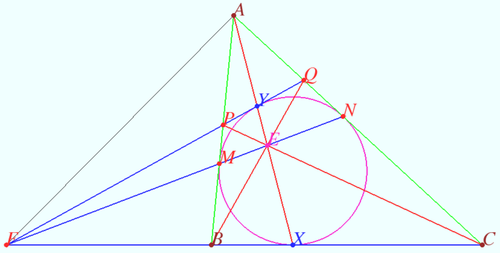
Solution 4 (Projective geometry)
Lemma
Let the sides ![]() and
and ![]() be tangent to
be tangent to ![]() at
at ![]() and
and ![]() , respectively. Then
lines
, respectively. Then
lines ![]() and
and ![]() are concurrent and lines
are concurrent and lines ![]() and
and ![]() are concurrent.
are concurrent.
Proof
Let ![]() be point of crossing
be point of crossing ![]() and
and ![]() We make projective transformation such that circle
We make projective transformation such that circle ![]() maps into the circle and point
maps into the circle and point ![]() maps into the center of new circle point
maps into the center of new circle point ![]() We denote images using notification
We denote images using notification ![]()
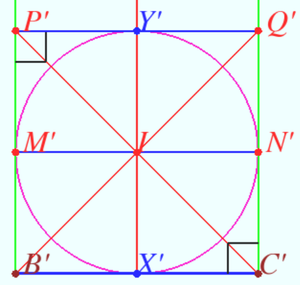
![]() maps into
maps into ![]() , so lines
, so lines ![]() and
and ![]() be the diameters.
This implies
be the diameters.
This implies ![]() be a square.
be a square.
Therefore ![]() be the diameter
be the diameter ![]() be diagonals of the square.
be diagonals of the square. ![]() and
and ![]() be midlines which crossing in the center
be midlines which crossing in the center ![]() Therefore ines
Therefore ines ![]() and
and ![]() are concurrent.
are concurrent.
Lines ![]() and
and ![]() are concurrent.
are concurrent.
Solution
The cross-ratio associated with a list of four collinear points ![]() is defined as
is defined as ![]() The cross-ratio be projective invariant of a quadruple of collinear points, so
The cross-ratio be projective invariant of a quadruple of collinear points, so
![]()
![]()
![]()
![]()
~vvsss, www.deoma-cmd.ru
See Also
| 2018 AIME II (Problems • Answer Key • Resources) | ||
| Preceded by Problem 13 |
Followed by Problem 15 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()