Difference between revisions of "1997 AIME Problems/Problem 6"
m |
(solution) |
||
| Line 1: | Line 1: | ||
== Problem == | == Problem == | ||
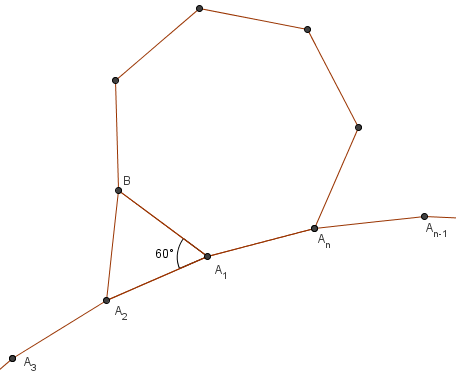
| − | Point <math>B</math> is in the exterior of the regular <math>n</math>-sided polygon <math>A_1A_2\cdots A_n</math>, and <math>A_1A_2B</math> is an equilateral triangle. What is the largest value of <math>n</math> for which <math>A_1</math>, <math>A_n</math>, and <math>B</math> are consecutive vertices of a regular polygon? | + | [[Point]] <math>B</math> is in the exterior of the [[regular polygon|regular]] <math>n</math>-sided polygon <math>A_1A_2\cdots A_n</math>, and <math>A_1A_2B</math> is an [[equilateral triangle]]. What is the largest value of <math>n</math> for which <math>A_1</math>, <math>A_n</math>, and <math>B</math> are consecutive vertices of a regular polygon? |
== Solution == | == Solution == | ||
| − | {{ | + | [[Image:1997_AIME-6.png]] |
| + | |||
| + | Let the other regular polygon have <math>m</math> sides. Using the interior angle of a regular polygon formula, we have <math>\angle A_2A_1A_n = \frac{(n-2)180}{n}</math>, <math>\angle A_nA_1B = \frac{(m-2)180}{m}</math>, and <math>\angle A_2A_1B = 60^{\circ}</math>. Since those three angles add up to <math>360^{\circ}</math>, | ||
| + | |||
| + | <cmath>\begin{eqnarray*} | ||
| + | \frac{(n-2)180}{n} + \frac{(m-2)180}{m} &=& 300\ | ||
| + | m(n-2)180 + n(m-2)180 &=& 300mn\ | ||
| + | 360mn - 360m - 360n &=& 300mn\ | ||
| + | mn - 6m - 6n &=& 0 | ||
| + | \end{eqnarray*}</cmath> | ||
| + | Using [[Simon's Favorite Factoring Trick|SFFT]], | ||
| + | |||
| + | <cmath>\begin{eqnarray*} | ||
| + | (m-6)(n-6) &=& 36 | ||
| + | \end{eqnarray*}</cmath> | ||
| + | Clearly <math>n</math> is maximized when <math>m = 7, n = \boxed{42}</math>. | ||
== See also == | == See also == | ||
{{AIME box|year=1997|num-b=5|num-a=7}} | {{AIME box|year=1997|num-b=5|num-a=7}} | ||
| + | |||
| + | [[Category:Intermediate Algebra Problems]] | ||
| + | [[Category:Intermediate Geometry Problems]] | ||
Revision as of 21:21, 21 November 2007
Problem
Point ![]() is in the exterior of the regular
is in the exterior of the regular ![]() -sided polygon
-sided polygon ![]() , and
, and ![]() is an equilateral triangle. What is the largest value of
is an equilateral triangle. What is the largest value of ![]() for which
for which ![]() ,
, ![]() , and
, and ![]() are consecutive vertices of a regular polygon?
are consecutive vertices of a regular polygon?
Solution
Let the other regular polygon have ![]() sides. Using the interior angle of a regular polygon formula, we have
sides. Using the interior angle of a regular polygon formula, we have ![]() ,
, ![]() , and
, and ![]() . Since those three angles add up to
. Since those three angles add up to ![]() ,
,
 Using SFFT,
Using SFFT,
![]() Clearly
Clearly ![]() is maximized when
is maximized when ![]() .
.
See also
| 1997 AIME (Problems • Answer Key • Resources) | ||
| Preceded by Problem 5 |
Followed by Problem 7 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||