Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2022 AIME II Problems/Problem 11"

(→‎Solution)
(→‎Solution)
Line 28: Line 28:
 
Finally, the square of the area is <math>(6\sqrt{5})^2=\boxed{180}</math>
 
Finally, the square of the area is <math>(6\sqrt{5})^2=\boxed{180}</math>
  
−
~DSAERF-CALMIT
+
~DSAERF-CALMIT (https://binaryphi.site)
  
 
==See Also==
 
==See Also==
 
{{AIME box|year=2022|n=II|num-b=10|num-a=12}}
 
{{AIME box|year=2022|n=II|num-b=10|num-a=12}}
 
{{MAA Notice}}
 
{{MAA Notice}}

Revision as of 00:29, 18 February 2022

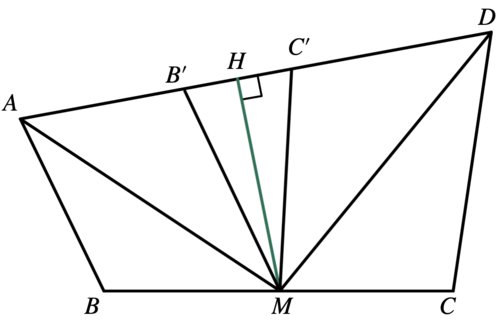
Problem

Let $ABCD$ be a convex quadrilateral with $AB=2$, $AD=7$, and $CD=3$ such that the bisectors of acute angles $\angle{DAB}$ and $\angle{ADC}$ intersect at the midpoint of $\overline{BC}$. Find the square of the area of $ABCD$.

Solution

2022 AIME II Q11(Hand-draw picture)


According to the problem, we have $AB=AB'=2$, $DC=DC'=3$, $MB=MB'$, $MC=MC'$, and $B'C'=7-2-3=2$

Because $M$ is the midpoint of $BC$, we have $BM=MC$, so: \[MB=MB'=MC'=MC.\]

Then, we can see that $\bigtriangleup{MB'C'}$ is an isosceles triangle with $MB'=MC'$

Therefore, we could start our angle chasing: $\angle{MB'C'}=\angle{MC'B'}=180^\circ-\angle{MC'D}=180^\circ-\angle{MCD}$.

This is when we found that points $M$, $C$, $D$, and $B'$ are on a circle. Thus, $\angle{BMB'}=\angle{CDC'} \Rightarrow \angle{B'MA}=\angle{C'DM}$. This is the time we found that $\bigtriangleup{AB'M} \sim \bigtriangleup{MC'D}$.

Thus, $\frac{AB'}{B'M}=\frac{MC'}{C'D} \Longrightarrow (BM')^2=AB' \cdot C'D = 6$

Point $H$ is the midpoint of $B'C'$, and $MH \perp AD$. $B'H=HC'=1 \Longrightarrow MH=\sqrt{B'M^2-B'H^2}=\sqrt{6-1}=\sqrt{5}$.

The area of this quadrilateral is the sum of areas of triangles: \[S_{\bigtriangleup{ABM}}+S_{\bigtriangleup{AB'M}}+S_{\bigtriangleup{CDM}}+S_{\bigtriangleup{CD'M}}+S_{\bigtriangleup{B'C'M}}\] \[=S_{\bigtriangleup{AB'M}}\cdot 2 + S_{\bigtriangleup{B'C'M}} + S_{\bigtriangleup{C'DM}}\cdot 2\] \[=2 \cdot \frac{1}{2} \cdot AB' \cdot MH + \frac{1}{2} \cdot B'C' \cdot MH + 2 \cdot \frac{1}{2} \cdot C'D \cdot MH\] \[=2\sqrt{5}+\sqrt{5}+3\sqrt{5}=6\sqrt{5}\]

Finally, the square of the area is $(6\sqrt{5})^2=\boxed{180}$

~DSAERF-CALMIT (https://binaryphi.site)

See Also

2022 AIME II (Problems • Answer Key • Resources)
Preceded by
Problem 10 		Followed by
Problem 12
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2022_AIME_II_Problems/Problem_11&oldid=171321"