Difference between revisions of "2007 AMC 12A Problems/Problem 6"
(→Solution 2) |
m (→Solution 1) |
||
| Line 8: | Line 8: | ||
[[Image:2007_AMC12A-6.png]] | [[Image:2007_AMC12A-6.png]] | ||
| − | We angle chase | + | We angle chase and find out that: |
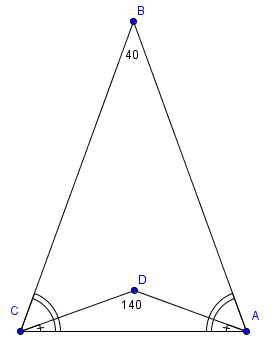
* <math>DAC=\frac{180-140}{2} = 20</math> | * <math>DAC=\frac{180-140}{2} = 20</math> | ||
* <math>BAC=\frac{180-40}{2} = 70</math> | * <math>BAC=\frac{180-40}{2} = 70</math> | ||
Revision as of 11:28, 3 June 2021
- The following problem is from both the 2007 AMC 12A #6 and 2007 AMC 10A #8, so both problems redirect to this page.
Contents
Problem
Triangles 
and 
are isosceles with 
and 
. Point 
is inside triangle , angle measures 40 degrees, and angle measures 140 degrees. What is the degree measure of angle 
?
Solution 1
We angle chase and find out that:
Solution 2
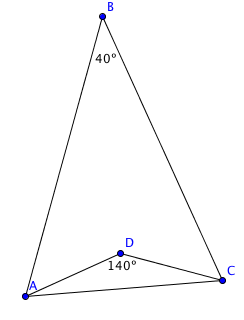
Since triangle is isosceles we know that angle 
.
Also since triangle is isosceles we know that 
.
This implies that 
.
Then the sum of the angles in quadrilateral 
is 
.
Solving the equation we get 
.
Therefore the answer is (D).
See also
| 2007 AMC 12A (Problems • Answer Key • Resources) | |
| Preceded by Problem 5 |
Followed by Problem 7 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
| 2007 AMC 10A (Problems • Answer Key • Resources) | ||
| Preceded by Problem 7 |
Followed by Problem 9 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. 
