Difference between revisions of "2005 AMC 10A Problems/Problem 23"
Dairyqueenxd (talk | contribs) (→Solution 4) |
Dairyqueenxd (talk | contribs) (→Solution 4) |
||
| Line 71: | Line 71: | ||
== Solution 4 == | == Solution 4 == | ||
| − | WLOG, let <math>AC=1</math>, <math>BC=2</math>, so radius of the circle is <math>\frac{3}{2}</math> and <math>OC=\frac{1}{2}</math>. As in Solution 1, By same altitude, the ratio <math>[DCE]/[ABD]=PE/AB</math>, where <math>P</math> is the point where <math>DC</math> extended meets circle <math>O</math>. Note that <math>\angle P = 90^{\circ}</math>, so <math>DCO | + | WLOG, let <math>AC=1</math>, <math>BC=2</math>, so radius of the circle is <math>\frac{3}{2}</math> and <math>OC=\frac{1}{2}</math>. As in Solution 1, By same altitude, the ratio <math>[DCE]/[ABD]=PE/AB</math>, where <math>P</math> is the point where <math>DC</math> extended meets circle <math>O</math>. Note that <math>\angle P = 90^{\circ}</math>, so <math>DCO \sim DPE</math> with ratio <math>1:2</math> so <math>PE = 1</math> Thus, our ratio is <math>\boxed{\textbf{(C) }\frac{1}{3}}</math>. |
== Solution 5 (Video) == | == Solution 5 (Video) == | ||
Revision as of 12:27, 14 December 2021
Contents
[hide]Problem
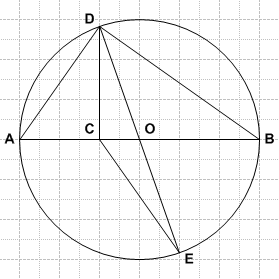
Let ![]() be a diameter of a circle and let
be a diameter of a circle and let ![]() be a point on
be a point on ![]() with
with ![]() . Let
. Let ![]() and
and ![]() be points on the circle such that
be points on the circle such that ![]() and
and ![]() is a second diameter. What is the ratio of the area of
is a second diameter. What is the ratio of the area of ![]() to the area of
to the area of ![]() ?
?
![[asy] unitsize(2.5cm); defaultpen(fontsize(10pt)+linewidth(.8pt)); dotfactor=3; pair O=(0,0), C=(-1/3.0), B=(1,0), A=(-1,0); pair D=dir(aCos(C.x)), E=(-D.x,-D.y); draw(A--B--D--cycle); draw(D--E--C); draw(unitcircle,white); drawline(D,C); dot(O); clip(unitcircle); draw(unitcircle); label("$E$",E,SSE); label("$B$",B,E); label("$A$",A,W); label("$D$",D,NNW); label("$C$",C,SW); draw(rightanglemark(D,C,B,2));[/asy]](http://latex.artofproblemsolving.com/a/8/0/a805b2766042251797b5817c889a0fb79a637874.png)
![]()
Solution 1
WLOG, Let us assume that the diameter is of length ![]() .
.
The length of ![]() is
is ![]() and
and ![]() is
is ![]() .
.
![]() is the radius of the circle, which is
is the radius of the circle, which is ![]() , so using the Pythagorean Theorem the height
, so using the Pythagorean Theorem the height ![]() of
of ![]() is
is  . This is also the height of the
. This is also the height of the ![]() .
.
The area of ![]() is
is ![]() =
= ![]() .
.
The height of ![]() can be found using the area of
can be found using the area of ![]() and
and ![]() as base.
as base.
Hence, the height of ![]() is
is ![]() =
= ![]() .
.
The diameter is the base for both the triangles ![]() and
and ![]() ,
,
Hence, the ratio of the area of ![]() to the area of
to the area of ![]() is
is
![]() =
= ![]()
Solution 2
Since ![]() and
and ![]() share a base, the ratio of their areas is the ratio of their altitudes. Draw the altitude from
share a base, the ratio of their areas is the ratio of their altitudes. Draw the altitude from ![]() to
to ![]() .
.
![[asy] import graph; import olympiad; pair O,A,B,C,D,E,F; O=(0,0);A=(15,0);B=(-15,0);C=(5,0);D=(5,14.142135623730950488016887242097);E=(-5,-14.142135623730950488016887242097);F=(0.5555555555555555,1.5713484026367722764463208046774); draw(Circle((0,0),15)); draw(A--B);draw(D--E);draw(C--D);draw(C--E);draw(C--F);draw(A--D);draw(D--B); label("A",A,NE);label("B",B,W);label("C",C,SE);label("D",D,NE);label("E",E,SW);label("O",O,SW);label("F",F,NW); markscalefactor=0.2; draw(anglemark(C,F,D),blue);draw(anglemark(D,C,B),blue); [/asy]](http://latex.artofproblemsolving.com/5/e/9/5e9629039a8bbef0413de3c19a864acbdb600acc.png)
![]() .
.
Since ![]() , then
, then ![]() . So the ratio of the two altitudes is
. So the ratio of the two altitudes is ![]()
Solution 3
Say the center of the circle is point ![]() ;
Without loss of generality, assume
;
Without loss of generality, assume ![]() , so
, so ![]() and the diameter and radius are
and the diameter and radius are ![]() and
and ![]() , respectively. Therefore,
, respectively. Therefore, ![]() , and
, and ![]() .
The area of
.
The area of ![]() can be expressed as
can be expressed as ![]()
![]() happens to be the area of
happens to be the area of ![]() . Furthermore,
. Furthermore, ![]() or
or ![]() Therefore, the ratio is
Therefore, the ratio is ![]()
Solution 4
WLOG, let ![]() ,
, ![]() , so radius of the circle is
, so radius of the circle is ![]() and
and ![]() . As in Solution 1, By same altitude, the ratio
. As in Solution 1, By same altitude, the ratio ![]() , where
, where ![]() is the point where
is the point where ![]() extended meets circle
extended meets circle ![]() . Note that
. Note that ![]() , so
, so ![]() with ratio
with ratio ![]() so
so ![]() Thus, our ratio is
Thus, our ratio is ![]() .
.
Solution 5 (Video)
Video solution: https://youtu.be/i6eooSSJF64
See Also
| 2005 AMC 10A (Problems • Answer Key • Resources) | ||
| Preceded by Problem 22 |
Followed by Problem 24 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()