Difference between revisions of "2021 AIME II Problems/Problem 14"

Revision as of 09:09, 30 May 2022

Problem

Let $\Delta ABC$ be an acute triangle with circumcenter $O$ and centroid $G$ . Let $X$ be the intersection of the line tangent to the circumcircle of $\Delta ABC$ at $A$ and the line perpendicular to $GO$ at $G$ . Let $Y$ be the intersection of lines $XG$ and $BC$ . Given that the measures of $\angle ABC, \angle BCA,$ and $\angle XOY$ are in the ratio $13 : 2 : 17,$ the degree measure of $\angle BAC$ can be written as $\frac{m}{n},$ where $m$ and $n$ are relatively prime positive integers. Find $m+n$ .

Diagram

$[asy] /* Made by MRENTHUSIASM */ size(375); pair A, B, C, O, G, X, Y; A = origin; B = (1,0); C = extension(A,A+10*dir(585/7),B,B+10*dir(180-585/7)); O = circumcenter(A,B,C); G = centroid(A,B,C); Y = intersectionpoint(G--G+(100,0),B--C); X = intersectionpoint(G--G-(100,0),A--scale(100)*rotate(90)*dir(O-A)); markscalefactor=3/160; draw(rightanglemark(O,G,X),red); dot("$A$",A,1.5*dir(180+585/7),linewidth(4)); dot("$B$",B,1.5*dir(-585/7),linewidth(4)); dot("$C$",C,1.5N,linewidth(4)); dot("$O$",O,1.5N,linewidth(4)); dot("$G$",G,1.5S,linewidth(4)); dot("$Y$",Y,1.5E,linewidth(4)); dot("$X$",X,1.5W,linewidth(4)); draw(A--B--C--cycle^^X--O--Y--cycle^^A--X^^O--G^^circumcircle(A,B,C)); [/asy]$ ~MRENTHUSIASM

Solution 1

In this solution, all angle measures are in degrees.

Let $M$ be the midpoint of $\overline{BC}$ so that $\overline{OM}\perp\overline{BC}$ and $A,G,M$ are collinear. Let $\angle ABC=13k,\angle BCA=2k$ and $\angle XOY=17k.$

Note that:

Since $\angle OGX = \angle OAX = 90,$ quadrilateral $OGAX$ is cyclic by the Converse of the Inscribed Angle Theorem.
It follows that $\angle OAG = \angle OXG,$ as they share the same intercepted arc $\widehat{OG}.$

Since $\angle OGY = \angle OMY = 90,$ quadrilateral $OGYM$ is cyclic by the supplementary opposite angles.
It follows that $\angle OMG = \angle OYG,$ as they share the same intercepted arc $\widehat{OG}.$

Together, we conclude that $\triangle OAM \sim \triangle OXY$ by AA, so $\angle AOM = \angle XOY = 17k.$

Next, we express $\angle BAC$ in terms of $k.$ By angle addition, we have $\begin{align*} \angle AOM &= \angle AOB + \angle BOM \\ &= 2\angle BCA + \frac12\angle BOC \hspace{10mm} &&\text{by Inscribed Angle Theorem and Perpendicular Bisector Property} \\ &= 2\angle BCA + \angle BAC. &&\text{by Inscribed Angle Theorem} \end{align*}$ Substituting back gives $17k=2(2k)+\angle BAC,$ from which $\angle BAC=13k.$

For the sum of the interior angles of $\triangle ABC,$ we get $\begin{align*} \angle ABC + \angle BCA + \angle BAC &= 180 \\ 13k+2k+13k&=180 \\ 28k&=180 \\ k&=\frac{45}{7}. \end{align*}$ Finally, we obtain $\angle BAC=13k=\frac{585}{7},$ from which the answer is $585+7=\boxed{592}.$

~Constance-variance (Fundamental Logic)

~MRENTHUSIASM (Reconstruction)

Solution 2

Let $M$ be the midpoint of $BC$ . Because $\angle{OAX}=\angle{OGX}=\angle{OGY}=\angle{OMY}=90^o$ , $AXOG$ and $OMYG$ are cyclic, so $O$ is the center of the spiral similarity sending $AM$ to $XY$ , and $\angle{XOY}=\angle{AOM}$ . Because $\angle{AOM}=2\angle{BCA}+\angle{BAC}$ , it's easy to get $\frac{585}{7} \implies \boxed{592}$ from here.

~Lcz

Solution 3 (Easy and Simple)

Firstly, let $M$ be the midpoint of $BC$ . Then, $\angle OMB = 90^o$ . Now, note that since $\angle OGX = \angle XAO = 90^o$ , quadrilateral $AGOX$ is cyclic. Also, because $\angle OMY + \angle OGY = 180^o$ , $OMYG$ is also cyclic. Now, we define some variables: let $\alpha$ be the constant such that $\angle ABC = 13\alpha, \angle ACB = 2\alpha,$ and $\angle XOY = 17\alpha$ . Also, let $\beta = \angle OMG = \angle OYG$ and $\theta = \angle OXG = \angle OAG$ (due to the fact that $AGOX$ and $OMYG$ are cyclic). Then, $\angle XOY = 180 - \beta - \theta = 17\alpha \implies \beta + \theta = 180 - 17\alpha.$ Now, because $AX$ is tangent to the circumcircle at $A$ , $\angle XAC = \angle CBA = 13\alpha$ , and $\angle CAO = \angle OAX - \angle CAX = 90 - 13\alpha$ . Finally, notice that $\angle AMB = \angle OMB - \angle OMG = 90 - \beta$ . Then, $\angle BAM = 180 - \angle ABC - \angle AMB = 180 - 13\alpha - (90 - \beta) = 90 + \beta - 13\alpha.$ Thus, $\angle BAC = \angle BAM + \angle MAO + \angle OAC = 90 + \beta - 13\alpha + \theta + 90 - 13\alpha = 180 - 26\alpha + (\beta + \theta),$ and $180 = \angle BAC + 13\alpha + 2\alpha = 180 - 11\alpha + \beta + \theta \implies \beta + \theta = 11\alpha.$ However, from before, $\beta+\theta = 180 - 17 \alpha$ , so $11 \alpha = 180 - 17 \alpha \implies 180 = 28 \alpha \implies \alpha = \frac{180}{28}$ . To finish the problem, we simply compute $\angle BAC = 180 - 15 \alpha = 180 \cdot \left(1 - \frac{15}{28}\right) = 180 \cdot \frac{13}{28} = \frac{585}{7},$ so our final answer is $585+7=\boxed{592}$ .

~advanture

Solution 4 (Guessing in the Last 3 Minutes, Unreliable)

Notice that $\triangle ABC$ looks isosceles, so we assume it's isosceles. Then, let $\angle BAC = \angle ABC = 13x$ and $\angle BCA = 2x.$ Taking the sum of the angles in the triangle gives $28x=180,$ so $13x = \frac{13}{28} \cdot 180 = \frac{585}{7}$ so the answer is $\boxed{592}.$

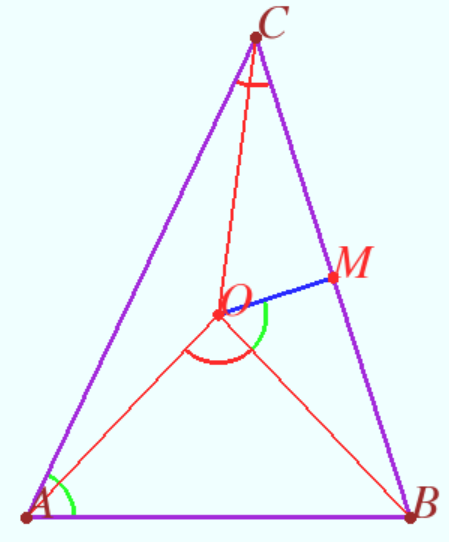
Solution 5 (Why isosceles)

Lemma:

Let $\triangle ABC$ be an acute triangle with circumcenter $O.$ Let $M$ be the midpoint of $BC$ so $MO\perp BC.$

If $\angle AOM = 2\angle ACB + \angle ABC,$ then $AC = BC.$

We define $\angle AOM$ as the sum of $\angle AOB + \angle BOM,$ this angle can be greater than $\pi.$

Proof:

$\angle BAC = \angle BOM$ as an inscribed and half central, based on the $\overset{\Large\frown} {BC}.$

$\angle AOB = 2\angle ACB$ as inscribed and central, based on the $\overset{\Large\frown} {AB}.$

$\angle AOM = \angle AOB + \angle BOM = 2 \angle ACB + \angle CAB.$

If $\angle AOM = 2 \angle ACB + \angle ABC,$ then $\angle ABC = \angle CAB, AC = BC.$

Solution:

Since $\angle OAX = \angle OGX = \frac {\pi}{2},$ quadrilateral $XAGO$ is cyclic by the Converse of the Inscribed Angle Theorem.

It follows that $\angle GXO = \angle GAO,$ as they share the same intersept $\overset{\Large\frown} {GO}.$ Since $\angle OGY = \angle OMY = \frac {\pi}{2},$ quadrilateral $OGYM$ is cyclic by the supplementary opposite angles.

It follows that $\angle GYO = \angle OMG,$ as they share the same intercept $\overset{\Large\frown} {GO}.$

In triangles $\triangle XOY$ and $\triangle AOM,$ two pairs of angles are equal, which means that the third angles $\angle XOY = \angle AOM$ are also equal. $\angle ABC : \angle BCA : \angle AOM = 13 : 2 : 17,$ so $\angle AOM = \angle ABC + 2 \angle BCA.$ According to the lemma, $\triangle ABC$ is isosceles, $\angle ABC : \angle BCA : \angle BAC = 13 : 2 : 13.$ $\angle BAC = \frac{13} {13 + 2 + 13} \cdot 180 = \frac {585}{7}.$

Video Solution 1

https://www.youtube.com/watch?v=zFH1Z7Ydq1s

~Mathematical Dexterity

Video Solution 2

https://www.youtube.com/watch?v=7Bxr2h4btWo

~Osman Nal

@@ Line 97: / Line 97: @@
 If  <math>\angle AOM = 2 \angle ACB +  \angle ABC,</math> then  <math>\angle ABC =  \angle CAB, AC = BC.</math>
+<pre style="color: blue">
+Solution:
+</pre>
+Since <math>\angle OAX = \angle OGX = \frac {\pi}{2},</math> quadrilateral <math>XAGO</math> is cyclic by the Converse of the Inscribed Angle Theorem.
+It follows that <math>\angle GXO =  \angle GAO,</math> as they share the same intersept  <math>\overset{\Large\frown} {GO}.</math>
+Since <math>\angle OGY = \angle OMY =  \frac {\pi}{2},</math> quadrilateral <math>OGYM</math> is cyclic by the supplementary opposite angles.
+It follows that <math>\angle GYO = \angle OMG,</math> as they share the same intercept <math>\overset{\Large\frown} {GO}.</math>
+In triangles <math>\triangle XOY</math> and  <math>\triangle AOM,</math> two pairs of angles are equal, which means that the third angles  <math>\angle XOY =  \angle AOM</math> are also equal.
+<math>\angle ABC :  \angle BCA :  \angle AOM = 13 : 2 : 17,</math> so  <math>\angle AOM =  \angle ABC + 2 \angle BCA.</math>
+According to the lemma,  <math>\triangle ABC</math> is isosceles,
+<cmath>\angle ABC : \angle BCA : \angle BAC = 13 : 2 : 13.</cmath>
+<cmath>\angle BAC = \frac{13} {13 + 2 + 13} \cdot 180 =  \frac {585}{7}.</cmath>
 ==Video Solution 1==

2021 AIME II (Problems • Answer Key • Resources)
Preceded by Problem 13	Followed by Problem 15
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

Page

Toolbox

Search