1961 AHSME Problems/Problem 11
Contents
[hide]Problem
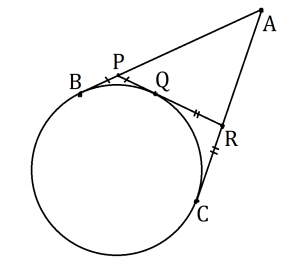
Two tangents are drawn to a circle from an exterior point ![]() ; they touch the circle at points
; they touch the circle at points ![]() and
and ![]() respectively.
A third tangent intersects segment
respectively.
A third tangent intersects segment ![]() in
in ![]() and
and ![]() in
in ![]() , and touches the circle at
, and touches the circle at ![]() . If
. If ![]() , then the perimeter of
, then the perimeter of ![]() is
is
![]()
Solution
Draw the diagram as shown. Note that the two tangent lines from a single outside point of a circle have the exact same length, so ![]() ,
, ![]() , and
, and ![]() .
.
The perimeter of the triangle is ![]() . Note that
. Note that ![]() , so from substitution, the perimeter is
, so from substitution, the perimeter is
![]()
![]()
![]() Thus, the perimeter of the triangle is
Thus, the perimeter of the triangle is ![]() , so the answer is
, so the answer is ![]() .
.
Solution 2 (Non-rigorous)
Since ![]() can be anywhere on the circle between
can be anywhere on the circle between ![]() and
and ![]() , it can basically be "on top" of
, it can basically be "on top" of ![]() . Then
. Then ![]() will be at the same point as
will be at the same point as ![]() , so
, so ![]() form a degenerate triable with side length
form a degenerate triable with side length ![]() . So its perimeter will be
. So its perimeter will be ![]() . Since
. Since ![]() and
and ![]() by power of a point, as
by power of a point, as ![]() and
and ![]() decrease in length,
decrease in length, ![]() will "grow" to compensate, so the perimeter will stay constant with a value of
will "grow" to compensate, so the perimeter will stay constant with a value of ![]() .
.
We can also skip verifying that the perimeter will stay constant, since it seems unlikely that MAA would create a question with ![]() as the answer.
as the answer.
See Also
| 1961 AHSC (Problems • Answer Key • Resources) | ||
| Preceded by Problem 10 |
Followed by Problem 12 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 • 26 • 27 • 28 • 29 • 30 • 31 • 32 • 33 • 34 • 35 • 36 • 37 • 38 • 39 • 40 | ||
| All AHSME Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()