Difference between revisions of "Euler line"
(→Euler line for a triangle with an angle of 120^\circ.) |
(→Euler line for a triangle with an angle of 120^\circ.) |
||
| Line 56: | Line 56: | ||
'''vladimir.shelomovskii@gmail.com, vvsss''' | '''vladimir.shelomovskii@gmail.com, vvsss''' | ||
| + | |||
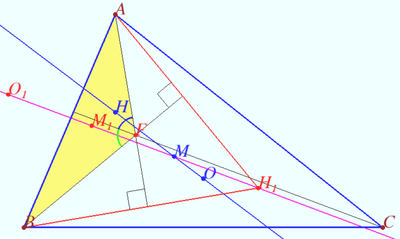
| + | ==Concurrent Euler lines and Fermat points== | ||
| + | [[File:Fermat point lines.png|400px|right]] | ||
| + | Consider a triangle <math>ABC</math> with Fermat–Torricelli points <math>F_1</math> and <math>F_2.</math> The Euler lines of the <math>10</math> triangles with vertices chosen from <math>A, B, C, F_1,</math> and <math>F_2</math> are concurrent at the centroid of triangle <math>ABC.</math> | ||
| + | |||
| + | <i><b>Case 1</b></i> | ||
| + | Let <math>F</math> be Fermat point <math>F_1</math> of <math>\triangle ABC</math> maximum angle of which smaller then <math>120^\circ.</math> Then the centroid of triangle <math>ABC</math> lies on Euler line of the <math>\triangle ABF.</math> | ||
==See also== | ==See also== | ||
Revision as of 03:51, 20 October 2022
In any triangle ![]() , the Euler line is a line which passes through the orthocenter
, the Euler line is a line which passes through the orthocenter ![]() , centroid
, centroid ![]() , circumcenter
, circumcenter ![]() , nine-point center
, nine-point center ![]() and de Longchamps point
and de Longchamps point ![]() . It is named after Leonhard Euler. Its existence is a non-trivial fact of Euclidean geometry. Certain fixed orders and distance ratios hold among these points. In particular,
. It is named after Leonhard Euler. Its existence is a non-trivial fact of Euclidean geometry. Certain fixed orders and distance ratios hold among these points. In particular, ![]() and
and ![]()
Euler line is the central line ![]() .
.
Given the orthic triangle ![]() of
of ![]() , the Euler lines of
, the Euler lines of ![]() ,
,![]() , and
, and ![]() concur at
concur at ![]() , the nine-point circle of
, the nine-point circle of ![]() .
.
Contents
Proof Centroid Lies on Euler Line
This proof utilizes the concept of spiral similarity, which in this case is a rotation followed homothety. Consider the medial triangle ![]() . It is similar to
. It is similar to ![]() . Specifically, a rotation of
. Specifically, a rotation of ![]() about the midpoint of
about the midpoint of ![]() followed by a homothety with scale factor
followed by a homothety with scale factor ![]() centered at
centered at ![]() brings
brings ![]() . Let us examine what else this transformation, which we denote as
. Let us examine what else this transformation, which we denote as ![]() , will do.
, will do.
It turns out ![]() is the orthocenter, and
is the orthocenter, and ![]() is the centroid of
is the centroid of ![]() . Thus,
. Thus, ![]() . As a homothety preserves angles, it follows that
. As a homothety preserves angles, it follows that ![]() . Finally, as
. Finally, as ![]() it follows that
it follows that
![]() Thus,
Thus, ![]() are collinear, and
are collinear, and ![]() .
.
Another Proof
Let ![]() be the midpoint of
be the midpoint of ![]() .
Extend
.
Extend ![]() past
past ![]() to point
to point ![]() such that
such that ![]() . We will show
. We will show ![]() is the orthocenter.
Consider triangles
is the orthocenter.
Consider triangles ![]() and
and ![]() . Since
. Since ![]() , and they both share a vertical angle, they are similar by SAS similarity. Thus,
, and they both share a vertical angle, they are similar by SAS similarity. Thus, ![]() , so
, so ![]() lies on the
lies on the ![]() altitude of
altitude of ![]() . We can analogously show that
. We can analogously show that ![]() also lies on the
also lies on the ![]() and
and ![]() altitudes, so
altitudes, so ![]() is the orthocenter.
is the orthocenter.
Proof Nine-Point Center Lies on Euler Line
Assuming that the nine point circle exists and that ![]() is the center, note that a homothety centered at
is the center, note that a homothety centered at ![]() with factor
with factor ![]() brings the Euler points
brings the Euler points ![]() onto the circumcircle of
onto the circumcircle of ![]() . Thus, it brings the nine-point circle to the circumcircle. Additionally,
. Thus, it brings the nine-point circle to the circumcircle. Additionally, ![]() should be sent to
should be sent to ![]() , thus
, thus ![]() and
and ![]() .
.
Analytic Proof of Existence
Let the circumcenter be represented by the vector ![]() , and let vectors
, and let vectors ![]() correspond to the vertices of the triangle. It is well known the that the orthocenter is
correspond to the vertices of the triangle. It is well known the that the orthocenter is ![]() and the centroid is
and the centroid is ![]() . Thus,
. Thus, ![]() are collinear and
are collinear and ![]()
Euler line for a triangle with an angle of 120
Let the ![]() in triangle
in triangle ![]() be
be ![]() Then the Euler line of the
Then the Euler line of the ![]() is parallel to the bisector of
is parallel to the bisector of ![]()
Proof
Let ![]() be circumcircle of
be circumcircle of ![]()
Let ![]() be circumcenter of
be circumcenter of ![]()
Let ![]() be the circle symmetric to
be the circle symmetric to ![]() with respect to
with respect to ![]()
Let ![]() be the point symmetric to
be the point symmetric to ![]() with respect to
with respect to ![]()
The ![]() lies on
lies on ![]() lies on
lies on ![]()
![]() is the radius of
is the radius of ![]() and
and ![]() translation vector
translation vector ![]() to
to ![]() is
is ![]()
Let ![]() be the point symmetric to
be the point symmetric to ![]() with respect to
with respect to ![]() Well known that
Well known that ![]() lies on
lies on ![]() Therefore point
Therefore point ![]() lies on
lies on ![]()
Point ![]() lies on
lies on ![]()
Let ![]() be the bisector of
be the bisector of ![]() are concurrent.
are concurrent.
![]()
Euler line ![]() of the
of the ![]() is parallel to the bisector
is parallel to the bisector ![]() of
of ![]() as desired.
as desired.
vladimir.shelomovskii@gmail.com, vvsss
Concurrent Euler lines and Fermat points
Consider a triangle ![]() with Fermat–Torricelli points
with Fermat–Torricelli points ![]() and
and ![]() The Euler lines of the
The Euler lines of the ![]() triangles with vertices chosen from
triangles with vertices chosen from ![]() and
and ![]() are concurrent at the centroid of triangle
are concurrent at the centroid of triangle ![]()
Case 1
Let ![]() be Fermat point
be Fermat point ![]() of
of ![]() maximum angle of which smaller then
maximum angle of which smaller then ![]() Then the centroid of triangle
Then the centroid of triangle ![]() lies on Euler line of the
lies on Euler line of the ![]()
See also
This article is a stub. Help us out by expanding it.