2007 Cyprus MO/Lyceum/Problem 21
Problem
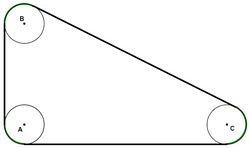
In the figure, three equal cycles of diameter ![]() represent pulleys, that are connected with a strap. If the distances between any two pulley center points are
represent pulleys, that are connected with a strap. If the distances between any two pulley center points are ![]() ,
, ![]() and
and ![]() , then the length of the strap is
, then the length of the strap is
![]()
Solution
The lengths of the straight parts of the strap are ![]() ,
, ![]() , and
, and ![]() . Their sum is
. Their sum is ![]() . The curved parts of the band add up to a full circumference of one of the circles, so their sum is
. The curved parts of the band add up to a full circumference of one of the circles, so their sum is ![]() . The total length of the strap is
. The total length of the strap is ![]() .
.
See also
| 2007 Cyprus MO, Lyceum (Problems) | ||
| Preceded by Problem 20 |
Followed by Problem 22 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 • 26 • 27 • 28 • 29 • 30 | ||