2007 Cyprus MO/Lyceum/Problem 22
Revision as of 01:19, 24 April 2008 by I like pie (talk | contribs)
Problem
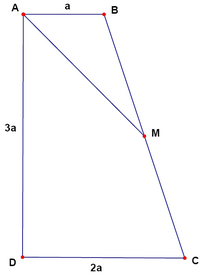
In the figure, ![]() is an orthogonal trapezium with $\ang A= \ang D=90^\circ$ (Error compiling LaTeX. Unknown error_msg) and bases
is an orthogonal trapezium with $\ang A= \ang D=90^\circ$ (Error compiling LaTeX. Unknown error_msg) and bases ![]() ,
, ![]() . If
. If ![]() and
and ![]() is the midpoint of the side
is the midpoint of the side ![]() , then
, then ![]() equals to
equals to
![]()
Solution
Let the midpoint of ![]() be
be ![]() . The length of
. The length of ![]() is the average of the bases, or
is the average of the bases, or ![]() . The length of
. The length of ![]() is also
is also ![]() .
.
Since ![]() is a
is a ![]() triangle, the length of
triangle, the length of ![]() is
is ![]() .
.
See also
| 2007 Cyprus MO, Lyceum (Problems) | ||
| Preceded by Problem 21 |
Followed by Problem 23 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 • 26 • 27 • 28 • 29 • 30 | ||