Difference between revisions of "1995 AIME Problems"
| Line 7: | Line 7: | ||
== Problem 2 == | == Problem 2 == | ||
| + | Find the last three digits of the product of the positive roots of | ||
| + | <math>\sqrt{1995}x^{\log_{1995}x}=x^2.</math> | ||
[[1995 AIME Problems/Problem 2|Solution]] | [[1995 AIME Problems/Problem 2|Solution]] | ||
Revision as of 21:55, 21 January 2007
Contents
[hide]Problem 1
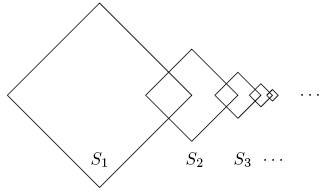
Square ![]() is
is ![]() For
For ![]() the lengths of the sides of square
the lengths of the sides of square ![]() are half the lengths of the sides of square
are half the lengths of the sides of square ![]() two adjacent sides of square
two adjacent sides of square ![]() are perpendicular bisectors of two adjacent sides of square
are perpendicular bisectors of two adjacent sides of square ![]() and the other two sides of square
and the other two sides of square ![]() are the perpendicular bisectors of two adjacent sides of square
are the perpendicular bisectors of two adjacent sides of square ![]() The total area enclosed by at least one of
The total area enclosed by at least one of ![]() can be written in the form
can be written in the form ![]() where
where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]()
Problem 2
Find the last three digits of the product of the positive roots of
![]()