Difference between revisions of "2002 AMC 12B Problems/Problem 14"
A1337h4x0r (talk | contribs) (→Solution 3) |
(Tag: Undo) |
||
| (14 intermediate revisions by 3 users not shown) | |||
| Line 19: | Line 19: | ||
==Solution 3== | ==Solution 3== | ||
| − | Pick a circle any circle- <math>4</math> ways. Then, pick any other circle- <math>3</math> ways. For each of these circles, there will be 2 intersections | + | Pick a circle any circle- <math>4</math> ways. Then, pick any other circle- <math>3</math> ways. For each of these circles, there will be <math>2</math> intersections for a total of <math>4*3*2</math> = <math>24</math> intersections. However, we have counted each intersection twice, so we divide for overcounting. Therefore, we reach a total of <math>\frac{24}{2}=\boxed{12}</math>, which corresponds to <math>\text{(D)}</math>. |
== See also == | == See also == | ||
Latest revision as of 13:04, 12 July 2021
- The following problem is from both the 2002 AMC 12B #14 and 2002 AMC 10B #18, so both problems redirect to this page.
Contents
[hide]Problem
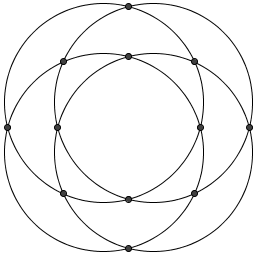
Four distinct circles are drawn in a plane. What is the maximum number of points where at least two of the circles intersect?
![]()
Solution 1
For any given pair of circles, they can intersect at most ![]() times. Since there are
times. Since there are  pairs of circles, the maximum number of possible intersections is
pairs of circles, the maximum number of possible intersections is ![]() . We can construct such a situation as below, so the answer is
. We can construct such a situation as below, so the answer is ![]() .
.
Solution 2
Because a pair or circles can intersect at most ![]() times, the first circle can intersect the second at
times, the first circle can intersect the second at ![]() points, the third can intersect the first two at
points, the third can intersect the first two at ![]() points, and the fourth can intersect the first three at
points, and the fourth can intersect the first three at ![]() points. This means that our answer is
points. This means that our answer is ![]()
Solution 3
Pick a circle any circle- ![]() ways. Then, pick any other circle-
ways. Then, pick any other circle- ![]() ways. For each of these circles, there will be
ways. For each of these circles, there will be ![]() intersections for a total of
intersections for a total of ![]() =
= ![]() intersections. However, we have counted each intersection twice, so we divide for overcounting. Therefore, we reach a total of
intersections. However, we have counted each intersection twice, so we divide for overcounting. Therefore, we reach a total of ![]() , which corresponds to
, which corresponds to ![]() .
.
See also
| 2002 AMC 10B (Problems • Answer Key • Resources) | ||
| Preceded by Problem 17 |
Followed by Problem 19 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
| 2002 AMC 12B (Problems • Answer Key • Resources) | |
| Preceded by Problem 13 |
Followed by Problem 15 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()