Difference between revisions of "2002 AMC 12B Problems/Problem 20"
m (→Solution) |
|||
| Line 22: | Line 22: | ||
<cmath>(2x)^2 + (2y)^2 = XY^2 \Longrightarrow XY = \sqrt{4(x^2 + y^2)} = \sqrt{4(169)} = \sqrt{676} = \boxed{\mathrm{(B)}\ 26}</cmath> | <cmath>(2x)^2 + (2y)^2 = XY^2 \Longrightarrow XY = \sqrt{4(x^2 + y^2)} = \sqrt{4(169)} = \sqrt{676} = \boxed{\mathrm{(B)}\ 26}</cmath> | ||
| + | |||
| + | Alternatively, we could note that since we found <math>x^2 + y^2 = 169</math>, segment <math>MN=13</math>. Right triangles <math>\triangle MON</math> and <math>\triangle XOY</math> are similar by Leg-Leg with a ratio of <math>\frac{1}{2}</math>, so <math>XY=2(MN)=\boxed{\mathrm{(B)}\ 26}</math> | ||
== See also == | == See also == | ||
Revision as of 12:13, 26 December 2016
- The following problem is from both the 2002 AMC 12B #20 and 2002 AMC 10B #22, so both problems redirect to this page.
Problem
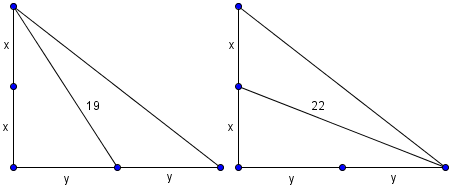
Let ![]() be a right-angled triangle with
be a right-angled triangle with ![]() . Let
. Let ![]() and
and ![]() be the midpoints of legs
be the midpoints of legs ![]() and
and ![]() , respectively. Given that
, respectively. Given that ![]() and
and ![]() , find
, find ![]() .
.
![]()
Solution
Let ![]() ,
, ![]() . By the Pythagorean Theorem on
. By the Pythagorean Theorem on ![]() respectively,
respectively,
![]()
Summing these gives ![]() .
.
By the Pythagorean Theorem again, we have
![]()
Alternatively, we could note that since we found ![]() , segment
, segment ![]() . Right triangles
. Right triangles ![]() and
and ![]() are similar by Leg-Leg with a ratio of
are similar by Leg-Leg with a ratio of ![]() , so
, so ![]()
See also
| 2002 AMC 10B (Problems • Answer Key • Resources) | ||
| Preceded by Problem 21 |
Followed by Problem 23 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
| 2002 AMC 12B (Problems • Answer Key • Resources) | |
| Preceded by Problem 19 |
Followed by Problem 21 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.